FOREWORD
RogerPenrose
Complexanalysisisthetheoryoffunctionsofcomplexnumbersor,morespecifically, holomorphic functionsofsuchnumbers.Thistheoryisbothprofoundly beautifulandvastlyinfluential,bothinpuremathematicsandinmanyareasof application,particularlyinphysics,indeedbeingcentraltotheunderlyingformalismofquantummechanics.However,theveryconceptofacomplexnumberisan essentiallyabstractone,dependingupontheseeminglyabsurdnotionofasquare rootof 1,thesquareofanyordinaryrealnumberbeing,unlike 1,necessarily non-negative.
Yet,itshouldbeborneinmindthateventhenotionofaso-called“real”numberisalsoanabstraction,andwemustmovefarbeyondtheimmediatenotionof “countingnumbers”0,1,2,3,4, ...,andbeyondeventhefractions 1 2 , 3 5 , 7 4 ,etc.,if wearetoexpresseventhesquarerootof2.Butherewearehelpedbya visualimage, andcanperceiveastraightlineextendedindefinitelyinbothdirectionstogiveus agoodintuitiveimpressionofthefullarrayofrealnumbers.Theslightlymisleadingterm“real”forthisimaginedarrayisexcusable,aswecanindeedimaginea ruler,oralineofinkdrawnonapieceofpaper,asprovidinguswithsomesortof conceptualimageofthisarray.Thisgreatlyhelpsourunderstandingsofwhatthe mathematician’sprecisenotionofa“realnumber”isintendedtoidealize.Weare notconcernedwithwhatevermightbethenatureofthephysicsoftheparticlesor fieldsthatmightcomposeourrulerorink-line;nor,indeed,dowerequireanyconceptofthecosmologythatmayberelevanttotheextensionouttoinfinityofour imaginedrulerorink-line.Ourabstractmathematicalnotionofa“realnumber” remainsalooffromanysuchrealitiesoftheactualworld.Yet,inacuriousreversal ofroles,itisthisverymathematicalidealizationthatunderliesmostofourtheories oftheactualworld.
So,whatcanbethedrivingforcebehindaneedtogobeyondtheseseemingly ubiquitous“realnumbers”?Whatpurposemighttherebefortheintroductionof a“squarerootof 1”?Suchanumberfitsnowherewithinthespanofthereal numbers,anditwouldappearthatwehavenoreasontodemandthattheequation x2 + 1 = 0haveanykindof“solution”.Theanswertothisdesireforsuchentities liesinthe magic thatlieshiddenwithinthem,butitisnotamagicthatimmediately revealsitself.Infact,whenthefirsthintofthismagicwasactuallyperceived,in
themid16th century,byGirolamoCardano,andthenmorecompletelybyRafael Bombelli,thesestrangenumbersweredismissed,evenbythem,asbeingasuseless astheyweremysterious.
Itisofsomeinteresttonotethatitwasnotintheequation x2 + 1 = 0thatthis hintofmagicwasfirstperceived,butincubicequationslike x3 = 15x + 4,which hastheperfectlysensiblesolution x = 4.Yet,asBombellihadnoted,Cardano’s generalexpressionforthesolutionofsuchcubicequationsnecessarilyinvolvesa detourintoamysteriousworldofnumbersofasortwheretheequation x2 + 1 = 0 isdeemedtohavethetwosolutions,nowreferredtoasthe imaginaryunits x = i and x =−i,ofanalgebra—nowcalled complex-number algebra—thathadappeared tobeconsistent,butnotwhathadbeenregardedas“real”.
Thisdismissiveattitudedidnotchangemuchuntilthemidtolate18th century,withLeonhardEuler’sremarkableformula eiθ = cos θ + i sin θ and,even moreimportantly,thegeometricalrepresentationoftheentirefamilyofcomplex numbersaspointsinaEuclideanplane,asinitiallyproposedbyCasparWessel, wherethealgebraicoperationsoncomplexnumbersarereadilyunderstoodin geometricalterms.Thisprovidedakindof2-dimensional“visualreality”tothe arrayofcomplexnumbersthatcouldbecombinedwithtopologicalnotions,such asemployedinitiallybyCarlFriedrichGauss,andsoonfollowedbyothers.The earlytomid19th centurysawmanyimportantadvances,manyofthesebeingdue toAugustin-LouisCauchy,especiallywiththebeautyandthepowerofcontour integration,and,perhapsmostprofoundly,withideasduetoBernhardRiemann. Theverynotionof“complexsmoothness”ofcomplexfunctionswasexpressed byuseoftheCauchy–Riemannequations,andthisprovidedthepowerfulconceptofa holomorphicfunction thatimpliesthatapower-seriesexpansionalways locallyexists,thisleadingtoavastandpowerfultheorywithnumerousmagical properties.
Thetworevolutionsofearly20th centuryphysicsbothoweaprofounddebt tocomplex-numbermathematics.Thisismostmanifestwithquantummechanics, sincethebasicformalismofthattheorydependsfundamentallyoncomplexnumbersandholomorphicfunctions.Weseearemarkableinterplaybetweenquantum spinandthegeometryofcomplexnumbers.ThebasicequationsofSchrödinger andDiracarebothcomplexequations.Inrelativitytheory,thetransformations relatingthevisualfieldoftwoobserverspassingclosebyeachotheratdifferentrelativisticspeedsismosteasilyunderstoodintermsofsimpleholomorphic functions.Moreover,manysolutionsofEinstein’sequationsforgeneralrelativity benefitgreatlyfrompropertiesofholomorphicfunctions,asdoesthedescription ofgravitationalwaves.
Inviewoftheundoubtedimportanceofcomplexanalysisinsomuchofmathematicsandphysics,itisclearlyimportantthattherearebasicaccountsofthese topicsavailabletothoseunfamiliar(oronlypartlyfamiliar)withthebasicideas
ofcomplexanalysis.InthisforewordIhaveverymuchstressedhowthevisual orgeometricviewpointhasbeenofvitalimportance,notonlytothehistorical developmentofcomplexanalysis,butalsototheproperunderstandingofthe subject.TristanNeedham’s VisualComplexAnalysis asoriginallypublishedin1997 was,tomyknowledge,uniqueintheextenttowhichitwasabletocoverthese fundamentalideaswithsuchthoroughness,visualelegance,andclarity.
Withthis 25thAnniversaryEdition therehavebeensomesignificantimprovements,mostparticularlyintheincorporationofcaptionstothediagrams.This makesiteasierforthereadertodipintothearguments,asillustratedsoelegantlyin suchwonderfullyexpressivepictures,withoutnecessarilyhavingtolookthrough tofindtherelevantportionofthetext.Inanycase,Iamsurethatreaders,overa broadrangeofrelevantknowledge—fromthosewithnopriorexperienceofcomplexanalysistothosealreadyexperts—willgaingreatlyfromthecharm,distinct originality,andvisualclarityoftheargumentspresentedhere.
Introduction
Mathematicalrealityexistsoutsidetheconfinesofspaceandtime,butbooksabout mathematicsdonot.Aquartercenturyafteritspublication,Iamgratefulthatan entirelynewgenerationofmathematiciansandscientistshascontinuedtoembrace VCA’sunorthodox,intuitive,and,aboveall, geometrical approachtoComplex Analysis.Tomarktheoccasion,OxfordUniversityPresshasgraciouslypermittedmetorevisitthework,resultinginthecreationofthissignificantlyimproved 25thAnniversaryEdition.
PrincipalChangesintheNewEdition
PerhapsIshouldbeginbynotingwhathas not changed.AsIshallelaboratebelow, backin1997,oneofmykeymathematicalinnovationswastheapplicationofNewton’sgeometricalmethodsfromthe Principia (now335yearsold)totheComplex AnalysisofCauchyandRiemann(nowmorethan200yearsold).Therefore,Ihave notseenanyneedtoupdatethemainbodyofthetext,whichhasremainedalmost unchanged,saveforafewadditionalcorrections.
AllofthechangestothisneweditionofVCA—bothinappearanceand substance—cameaboutasadirectresultoftheknowledgeIgainedcreatingmysecondandfinalbook,1 VisualDifferentialGeometryandForms:AMathematicalDrama inFiveActs,PrincetonUniversityPress,2021(hereafterabbreviatedtoVDGF).In particular,IthankWandaEspañaofPUPforherbeautifulworkonthedesignof VDGF,forthisinturninspiredsomedesignelementsthatIrequestedofOUPfor thisneweditionofVCA.OfcourseIalsothankOxfordUniversityPressfor accepting someofthesesuggestions,whilealsoimprintingtheirowndistinctivestyleon thework.
• Themostobviouschangeisinthephysicaldimensionsofthebook:ithas expandedfrom6′′×9′′ to7′′×10′′.Therearetwosignificantadvantagesto thischange:(1)thebookismorecomfortabletoholdandread;(2)the5032
1 Needham (2021).
2 Theoriginaleditiononlycontained501figures:ThefigureinthisPrefaceand[6.41]arebothnew.
figures—thebeatingheartofthework!—arenow36%larger,and,onemaydare tohope,36%clearer!
• Anotherobviouschangeistheintroductionofthestandardnumberingsystemforsections,subsections,equations,andfigures.Inhindsight,mydeliberate avoidanceofthestandardschemewasperhapsaratherchildish,tantrum-like expressionofmydisgustwiththeprevailing,life-suckingreductionofwildly excitingmathematicalideastothearidstructureenshrinedintraditionaltreatises:“Lemma12.7.2impliesTheorem14.3.8”.Letmetakethisopportunityto apologize—better25yearslatethannever?—toeveryprofessorwhowasever braveenoughtoadoptVCAasthetextfortheircourse,onlytodiscoverthat theyhadtostruggletorefertheirstudentstoanygivenfigureorresult!
• The Bibliography hasbeenupdated.Notonlyhavepreviouslycitedworksbeen updatedtotheirlatesteditions,butIhavealsoaddedanumberof new works, whichwerenotcitedinthefirstedition,forthesimplereasonthat25yearsago theydidnot exist!
• The Index hasbeenimprovedsignificantly.Manynewentrieshavebeenadded, and,perhapsmoreimportantly,whereverasinglemainentryformerlylisted along,frustratinglyunhelpfulstringofundifferentiatedpagenumbers,Ihave nowsplititintomanyindividualand helpfullyspecificsubentries.Bywayofproof, considertheentryfor“Jacobianmatrix”,thenandnow!
• GivingcredittoEugenioBeltrami.IntheoriginaleditionIpointedoutthatin1868 Beltramidiscovered3 (andpublished)theconformalmodelsofthehyperbolic plane,whichPoincaréthenrediscovered14yearslater,in1882.Now,following theexampleIsetmyselfinVDGF,Ihavegoneonestepfurther,attemptingtoput therecordstraightby renaming thesemodelsasthe Beltrami–Poincaré discand half-plane.Beltramialsodiscoveredtheprojectivemodel,andIhaverenamed that,too,asthe Beltrami–Klein model.Correspondingly,myfancifullynamed inhabitantsofthesehyperbolicworldshavebeenrenamedfrom“Poincarites” to Beltrami–Poincarites!
• Twentyfiveyearsago,VCAwasonthebleedingedgeofwhatwas typographically possible.Indeed,myeditortoldmethatVCAwasthe first mathematics bookpublishedbyOxfordUniversityPresstobecomposedinLATEXandyet not tobetypesetusingthestandardComputerModernfontsdesignedbyDonald Knuth,thecreatorofTEX.IwasabletoachievethisfeatbyvirtueofMichael Spivak’s4 thennewlycreated MathTime typeface,andbyvirtueofthewonderful
3 See Milnor (1982), Stillwell (1996),and Stillwell (2010).
4 Yes,thefamousdifferentialgeometer!
Y&YTEXSystemforWindows. 5 IwastherebyabletotypesetthebookinTimes textand(mainly)MathTimemathematics—a vast aestheticimprovementover ComputerModern,inmyhumblebutstrongopinion!
Needlesstosay,the TEX worldhasmovedonconsiderablyinthepast25years. Butinordertotakeadvantageoftheseadvances,Iwasforcedtograpplewith thetaskofupdatingmyoriginal TEX files(includingmycountlessmacros)from theancient(dead?)languageof LATEX2.09tothemodernLATEX2ε.Thiseffortwas rewardedwiththeabilitytotypesetthisneweditionofVCAusingthesametypefacesthatIverycarefullyselectedforVDGF,allthreeofwhichsprangfromthe geniusmindofHermannZapf(1918–2015):Optimafortheheadings,Palatinofor thetext,and,crucially,theremarkableEulerfontsforthe mathematics. LetmepauseforamomenttopaytributetoZapf’sEulermathematicalfonts. Theywouldappeartometobeathomeonboardthestarshipofanadvancedalien civilization,yettheyalsoevokethetime-wornstoneengravingswithinanancient Greekruin—theirbeautytranscendsspaceandtime:Pictureaninscriptiononthe GuardianofForever!6
ButmyfatalattractiontothesefontsbroughtaboutconflictbetweentheEuler mathematicsinthetextoftheneweditionandthe MathTime mathematicsinall the figures oftheoriginaledition.Havingbeenborncursed(andIsupposeblessed) withacompulsiontostriveforperfection,Ihadnochoicebuttoundertakethe self-inflicted,Herculeantaskofhand-editing(withinCorelDRAW)all501ofmy original,hand-drawnfigures!IthenoutputnewversionsinwhicheachMathTime symbolisherereplacedwithitsmatchingEulercounterpart,therebybringingthe figuresintoperfectalignmentwiththenewtext.
Iamtheimpossiblyproudfatherofremarkabletwindaughters,FaithandHope. IamalsothefatherofVCAandofVDGF.Itisthereforeasourceofdeep,resonating joythat both setsoftwinsnow look liketwins!
• Nowletmeexplainthemostfundamentalchange,theonethattookmethe greatesttimeandefforttoaccomplish,andtheonethatIbelievetransformsthis 25thAnniversaryEdition intoatruly new edition,onethatmayevenbeofvalue toownersoftheoriginaledition.
The503figuresarethemathematical soul ofthework.Theycrystallizeallthe geometricalinsightsIwasabletogleanfrommymanyyearsspentstrugglingto understand ComplexAnalysis.Yet,intheentireoriginaledition, youwillnotfinda single caption.Why?!
Sadly,theanswerissimple: cowardice.AsanewlymintedDPhilstudentofPenrose,withnotrackrecordorreputation,Ifearedthatthemathematicalcommunity
5 Sadly,thissystem(designedbyProfessorBertholdHornofMIT)ceasedtoexistin2004.
6 Thespace–timeportalfeaturedinthe StarTrek episode,“TheCityontheEdgeofForever”.
wouldrejectorevenridicule7 myNewtonianarguments.Ithereforesoughttocontrolthenarrative(literally!)byonlyrevealingtheargumentswithinthetextproper, whereIcouldspelloutmyreasoninginfull.
Well,25yearslater,Iamcertainlyolder,perhapsatadwiser,andIhavealso gainedamodicumofconfidencebyvirtueoftheenthusiasticreviewsthatVCAhas sincereceivedinallthemajorjournals.Perhapsmoreemotionallysignificanttome hasbeenthelargenumberof individual notesofappreciationIhavereceived,which Icontinuetoreceivetothisday,fromreadersofallstripes—graduatestudents, professors,andworkingscientists—fromaroundtheglobe.
So,finally,IhavedonesomethingthatIshouldhavehadthecouragetodoat theoutset:
Inthis 25thAnniversaryEdition, everyfigurenowhasa caption thatfullyexplainsitsmathematicalcontent.Additionally,manyof thesenewcaptionsincludea title (inboldprint)thatfurtherdistils thefigure’smeaningdowntoitsessence.
Thisapproach8 isdirectlyinspiredbytheworksofmyteacher,friend,andmentor, SirRogerPenrose—towhomthisbookisdedicated,andtowhomInowoffersincerethanksforhisgenerousForeword!—mostremarkablyinhis RoadtoReality, 9 whereasinglefigure’scaptioncantakeupaquarterofapage!
ThisinnovationnowmakesitpossibletoreadVCAinanentirelynewway—as ahighbrowcomicbook! Much ofthegeometricalreasoningoftheworkcannowbe graspedsimplybystudyingafigureanditsaccompanyingcaption,onlyturning tothemaintextforthecompleteexplanationasneeded.Furthermore,insteadof undertakingasystematic,linearreadingofthework,youarenowinvitedtoskip andhopabout,lightinguponwhicheverfigurehappenstocatchyoureye.
• Newton’sconceptof ultimateequality underliesmanyoftheargumentsinthis book,butitsusewasnotmade explicit intheoriginaledition,andthisledsome tosupposethattheargumentswerelessrigorousthantheyactuallywere(and remain).InthisneweditionIhaveonlyoccasionallymodifiedthemaintext,but I have feltatlibertytoexplicitlyintroduceultimateequalitiesintosomeofthe newlyminted captions.Asinmyearlierworks,10 Ihaveusedthesymbol ≍ to denotethisconcept.
Letmenowexpanduponthisvitallyimportantpoint ...
7 Inmydefence,suchfearswerenotentirelygroundless:WhenPrincetonUniversityPresssentout draftchaptersofVDGFforreview,oneofthethreeanonymousreviewersbluntlydeclared,“Thisis notevenmathematics!”
8 ThisisalsomyapproachinVDGF.
9 Penrose (2005).
10 Needham (1993), Needham (2014).
MoreonNewton’s Principia andHisConceptof UltimateEquality
AsIexplainintheoriginalPreface(immediatelyfollowingthisnewPreface),VCA (andVDGF)couldneverhavecometobehadInotundertakenacarefulstudy ofNewton’s Principia in1982,whileIwasstillaDPhilstudentofPenrose.AsI struggledtopenetrateNewton’sancientdiagrams,Iintuitivelysensedthepower andbeautyofhisgeometricalreasoning,longbeforeIfullyunderstoodit.
ButoneaspectofNewton’sthinkingdidmakesensetome immediately:why shouldNewtonsettleforstudyingan equation describingtheorbitofaplanet aroundtheSunwhenhepossessedageometricaltoolofenormouspower,capableofinsteadanalyzingtheorbit itself ?!Thisinturnprovokedwithinmea life-changing mathematicalcrisisofconscience:howmanyothermathematicalphenomenahadIfooledmyselfinto pretending Ihadunderstood,whenallIhaddone wasgraspanequationthat described thephenomenonfromafar,ratherthandaring tostaremathematicalrealitysquarelyand geometrically intheface?
Ifeltexcitedandstrangelyfearful,likeoneoftheapesintheopeningofStanley Kubrick’s, 2001:ASpaceOdyssey,shriekingandwildlyleapingaboutinthestark silentpresenceoftheblackmonolith.Iwantedtobethatoneapethatwasbrave enoughto touch themonolith,nowtakingtheformofadifferentancientreservoir ofdeeplyalienknowledge:Newton’smind!
SoIdid.AndonceIhaddoneso,IstareddownatNewton’sgeometricalconceptof ultimateequality,astheapehadstareddownatthelargefemurboneon thegroundbeforehim,pickeditup,gingerlyatfirst,examineditclosely,andthen spentthenext35yearsofmylifewieldingitwithalltheforceandingenuityI couldmuster—atooltocrackopenandlaybaretoourvisualintuitionthesecrets ofComplexAnalysis,and,25yearslater,thesecretsofDifferentialGeometryand Forms.
ThepresentationofthegeometricalreasoninginVDGF appears tobemorerigorousthanthatinVCAbecausethereI explicitly makeuseofNewton’sconceptof ultimateequality.LetmenowstealanexcerptfromthePrologueofVDGFinorder toexplainwhatthismeans.
AsIhavediscussedelsewhere,11 Newtonianscholarshavepainstakingly dismantledtheperniciousmyth12 thattheresultsinthe1687 Principia werefirst derivedbyNewtonusinghisoriginal1665versionofthecalculus,andonlylater recastintothegeometricalformthatwefindinthefinishedwork.
Instead,itisnowunderstoodthatbythemid-1670s,havingstudiedApollonius, Pappus,andHuygens,inparticular,thematureNewtonbecamedisenchantedwith
11 See Needham (1993),theoriginal1997PrefacetoVCA,and Needham (2014).
12 Sadly,thismythoriginatedwithNewtonhimself,intheheatofhisbitterprioritybattlewith Leibnizoverthediscoveryofthecalculus.See Arnol’d (1990), BloyeandHuggett (2011), deGandt (1995), Guicciardini (1999), Newton (1687,p.123),and Westfall (1980).
theforminwhichhehadoriginallydiscoveredthecalculusinhisyouth—which isdifferentagainfromtheLeibnizianformwealllearnincollegetoday—andhad insteadembracedpurelygeometricalmethods.
Thusitcametopassthatbythe1680sNewton’salgebraicinfatuationwithpower seriesgavewaytoanewformofcalculus—whathecalledthe“syntheticmethod offluxions”13—inwhichthegeometryoftheAncientswastransmogrifiedand reanimatedbyitsapplicationtoshrinkinggeometricalfiguresintheirmomentof vanishing. This isthepotentbutnon-algorithmicformofcalculusthatwefindin fullflowerinhisgreat Principia of1687.
LetmenowimmediatelyspelloutNewton’sapproach,andinsignificantly greaterdetailthanIdidinthefirsteditionofVCA,inthevainhopethatthis neweditionmaybolstermyeffortsinVDGFtoinspiremoremathematiciansand physiciststoadoptNewton’sintuitive(yetrigorous14)methodsthandidthefirst edition.
Iftwoquantities A and B dependonasmallquantity ϵ,andtheirratio approachesunityas ϵ approacheszero,thenIshallavoidthemorecumbersome languageoflimitsbyfollowingNewton’sprecedentinthe Principia,sayingsimply that,“A isultimatelyequalto B”.Also,asIdidinearlierworks[(Needham, 1993), (Needham, 2014)],Ishallemploythesymbol ≍ todenotethisconceptof ultimate equality. 15 Inshort,
A isultimatelyequalto B” ⇐⇒
Itfollows[exercise]fromthetheoremsonlimitsthatultimateequalityisanequivalencerelation,andthatitalsoinheritsadditionalpropertiesofordinaryequality, e.g.,
BeforeIbegintoapplythisideainearnest,Ialsonotethatthejurisdictionof ultimateequalitycanbeextendednaturallytothingsotherthannumbers,enabling onetosay,forexample,thattwotrianglesare“ultimatelysimilar”,meaningthat theiranglesareultimatelyequal.
AsIexplainintheoriginalPreface,havinggraspedNewton’smethod,Iimmediatelytriedmyownhandatusingittosimplifymyteachingofintroductory calculus,onlylaterrealizinghowImightapplyittoComplexAnalysis(inVCA), andlaterstilltoDifferentialGeometry(inVDGF).ThoughImightchooseanynumberofsimple,illustrativeexamples[see Needham (1993)formore],Iwillreusethe specificoneIgaveintheoriginalPrefacetoVCA,andforonesimplereason: this
13 See Guicciardini (2009,Ch.9).
14 Fineprinttofollow!
15 Thisnotationwassubsequentlyadopted(withattribution)bytheNobelphysicist,Subrahmanyan Chandrasekhar(see Chandrasekhar, 1995,p.44).
timeIwillusethe“≍”–notationto present theargumentrigorously,whereasinthe firsteditionIdidnot.Indeed,thisexamplemaybeviewedasarecipefortransformingmanyofVCA’s“explanations”into“proofs”,16 merelybysprinklingon therequisite ≍’s.Withtheadditionoffigurecaptions,Iamnowabletodosomeof thissprinklingmyself,butsomemuststillbelefttothereader.
Beforelookingatthisexample,Isuggestyoufirstreadtheoriginalpresentation oftheargumentintheoriginalPreface(immediatelyfollowingthisone)and then returntothispoint.
Letusshowthatif T = tan θ,then dT
= 1 + T 2 (seefigure).Ifweincrease θ byasmall(ultimatelyvanishing)amount δθ,then T willincreasebythelengthof theverticalhypotenuse δT ofthesmalltriangle,inwhichtheothertwosidesof thistrianglehavebeenconstructedtolieinthedirections (θ + δθ) and (θ + π 2 ),as illustrated.Toobtaintheresult,wefirstobservethatinthelimitthat δθ vanishes, thesmalltrianglewithhypotenuse δT isultimatelysimilartothelargetriangle withhypotenuse L,because ψ ≍ π 2 .Next,asweseeinthemagnifyingglass,the side δs adjacentto θ inthesmalltriangleisultimatelyequaltotheillustratedarc ofthecirclewithradius L,so δs ≍ Lδθ;notethatwehavemovedthedotfromthe cornerofthetriangletothearc,tostressthispoint.Thus,
SofarasIknow,Newtonneverwrotedownthisspecificexample,butcompare theilluminatingdirectnessofhis style ofgeometricalreasoningwiththeunilluminatingcomputationsweteachourstudentstoday,morethanthreecenturieslater! AsNewtonhimselfputit,17 thegeometricmethodistobepreferredbyvirtueof the“clarityandbrevityofthereasoninginvolvedandbecauseofthesimplicityof theconclusionsandtheillustrationsrequired.”Indeed,Newtonwentevenfurther, resolvingthat only thesyntheticmethodwas“worthyofpublicutterance”.
16 Iwasalreadyusingthe ≍ notation(bothprivatelyandinprint)atthetimeofwritingVCA,and, inhindsight,itwasamistakethatIdidnotemployitthroughouttheoriginaleditionofVCA.
17 See Guicciardini (2009,p.231)
Newtonhimselfdidnotemploy any symboltorepresenthisconceptof“ultimate equality”.Instead,hisdevotiontothegeometrical method oftheAncientsspilled overintoemulatingtheir mode ofexpression,causinghimtowriteoutthewords “ultimatelyhavetheratioofequality”everysingletimetheconceptwasinvoked inaproof.As Newton (1687,p.124)explained,the Principia is“writteninwordsat lengthinthemanneroftheAncients”.EvenwhenNewtonclaimedthattworatios wereultimatelyequal,heinsistedonexpressing eachratio inwords.Asaresult,I myselfwasquiteunabletofollowNewton’sreasoningwithoutfirsttranscribing andsummarizingeachofhisparagraphsinto“modern”form(whichwasinfact alreadyquitecommonin1687).Indeed,backin1982,thiswasthecatalystformy privateintroductionanduseofthesymbol ≍
ItismyviewthatNewton’schoice not tointroduceasymbolfor“ultimate equality”wasatragicallyconsequentialerrorforthedevelopmentofmathematics.AsLeibniz’ssymboliccalculusswepttheworld,Newton’smorepenetrating geometricalmethodfellbythewayside.IntheinterveningcenturiesonlyahandfulofpeopleeversoughttorepairthisdamageandreviveNewton’sapproach, themostnotableanddistinguishedrecentchampionhavingbeenV.I.Arnol’d18 [1937–2010].
HadNewtonshedthetrappingsofthisancientmodeofexpositionandinstead employedsomesymbol(any symbol!)inplaceofthewords“ultimatelyhavethe ratioofequality”,hisdense,paragraph-lengthproofsinthe Principia mighthave beenreducedtoafewsuccinctlines,andhismodeofthoughtmightstillbewidely employedtoday.BothVCAandVDGFareattemptstodemonstrate,veryconcretely,thecontinuingrelevanceandvitalityofNewton’sgeometricalapproach, inareasofmathematicswhosediscoverylayacenturyinthefutureatthetimeof hisdeathin1727.
Allowmetoinsertsomefineprintconcerningmyuseofthewords“rigour” and“proof”.Yes,myoccasionalexplicituseofNewtonianultimateequalitiesin thisneweditionrepresentsaquantumjumpinrigour,ascomparedtomyoriginal expositioninVCA,buttherewillbesomemathematicianswhowillobject(with justification!)thateventhisincreaseinrigourisinsufficient,andthat none ofthe “proofs”inthisworkareworthyofthattitle,includingtheonejustgiven:Idid notactuallyprovethatthesideofthetriangleisultimatelyequaltothearcofthe circle.
Icanofferno logical defence,butwillmerelyrepeatthewordsIwroteinthe originalPrefacetoVCA,25yearsago:“...supposeonebelieves,asIdo,thatour mathematicaltheoriesareattemptingtocaptureaspectsofarobustPlatonicworld thatisnotofourmaking.Iwouldthencontendthataninitiallackofrigourisa smallpricetopayifitallowsthereadertoseeintothisworldmoredirectlyand
18 See,forexample, Arnol’d (1990).
pleasurablythanwouldotherwisebepossible.”So,topreemptivelyaddressmy critics,letmethereforeconcede,fromtheoutset,thatwhenIclaimthatanassertion is“proved”,itmaybereadas, “provedbeyondareasonabledoubt”!19
TheContinuedRelevanceoftheUnorthodox Contents ofVCA
Leavingasideitsunorthodoxgeometrical methods,VCAisalsodistinguishedby itsunorthodox contents.Theconceptofthecomplexderivative(or amplitwist,asI callit)isnotevenintroduceduntilChapter4,andmanyofthemostinteresting, unusual,andimportantpartsofthebookmakelittleornouseofanalysis.
FormanydecadesbeforeVCA,andnowfordecadesafterVCA,almostall20 introductionstoComplexAnalysishaveseemedtofollowalmostexactlyinthe footstepsoftheonesthatcamebefore—thesametopics,explainedinthesame order,andinthesamemanner,likeasingle-fileprocessionofmonksthroughthe snow,quietlyandsombrelyintentonsteppingintothefootprintsofthemonkwho wentbeforethem:Donotdisturbthepristinesnowlyingallaboutyou!ButwhenI embarkeduponVCA,35yearsago,Iwaslikeahappilydisobedientchild,jumping aboutwildly,rollingmywholemindinthesnow!
Istartedfromscratch,askingmyself, whichideasconnectedtoComplexAnalysis havebecomethemostvitaltomodernmathematicsandphysics? Apairofcloselyrelated answerstothisquestionimmediatelypresentedthemselves,andwhileImakeno claimtoprescience,itiscertainlytruethatthefollowinghaveonlybecome more vitaltomathematicsandphysicsoverthepast25years:
• HyperbolicGeometry violatesthenormalrulesofEuclideangeometry,andit isthereforealsocalled Non-EuclideanGeometry.Inthehyperbolicplanethereare infinitelymanydifferentlinesthroughagivenpointthatareparalleltoagiven line,andtheanglesinatrianglealwaysaddupto less than π,thedeparturefrom π beingproportionaltothe area ofthetriangle.
Yet,asPoincaréwasthefirsttorecognize,thisstrangegeometryarisesnaturally acrossmanypartsofmathematicsandphysics.Forexample,thefinalfigureofthis bookrevealshowhyperbolicgeometryunifies all themethodsofsolvingthetwodimensionalDirichletProblem.Furthermore,thevisionaryinsightsofThurston21
19 Uponreadingthesewords,astronglysupportivememberoftheEditorialBoardofPrinceton UniversityPresssuggestedtomyeditorthatinplaceof“Q.E.D.”,Iconcludeeachofmyproofsin VDGFwiththeletters,“P.B.R.D.”!
20 IfreelyadmitthatIhavenotundertakenasystematicstudyofalltheComplexAnalysistextbooks thathavebeenpublishedduringthelast25years,andIamawarethatthereexistexcellent exceptions to thefollowinggeneralization,myfavouriteonesbeing Shaw (2006)and StewartandTall (2018),which Ihighlyrecommendprecisely because oftheirveryunusualcontents.Incidentally,Shawjusthappens tohavebeenafellowstudentofPenrose!
21 FordetailsofThurston’s GeometrizationConjecture—now Theorem!—see Thurston (1997).
(1946–2012)—subsequentlyvindicatedbyPerelmanin2003,sixyearsafterthepublicationofVCA—haveestablishedthathyperbolicgeometryisinsomesensemore fundamental thanEuclideangeometry.
Thisfascinatingandimportantgeometryisintimatelyentwinedwiththe complex numbers,viatheMöbiustransformationsthatweshalldiscussnext.Yet,aftera quartercentury,VCA’slongChapter6remainsthemostcompleteandthemost geometrical treatmentofhyperbolicgeometrythatIhaveseeninanyintroduction toComplexAnalysis.
• MöbiusTransformations aremappingsoftheform
ÞÑ M(z)= az + b cz + d , where a, b, c, d arecomplexconstants.Whereashyperbolicgeometryisrarely evenmentionedinintroductorytextsonComplexAnalysis,thesetransformationsarediscussedin all ofthem,butusuallyinaperfunctoryandsuperficial manner.Instarkcontrast,mylongChapter3remainsthemostcompleteand themost geometrical treatmentofthesetransformationsthatIhaveseeninany introductorytext.
ThereasonIlavishedsuchextravagantattentionuponthesedeceptivelysimpletransformationsisthattheyarepossessedof magical powers,manifesting themselvesinmultifacetedguisesacrossmathematicsandphysics.Again,itwas Poincaréwhowasthefirsttorecognizethis.Theyaretheisometriesofboththe twodimensionalhyperbolicplane(introducedabove)andof three-dimensionalhyperbolicspace;theyarethefamous Lorentztransformations (isometries)ofMinkowski andEinstein’s four-dimensionalspacetime;andwhentheconstantsareallintegers, and ad bc = 1,theyformthe modulargroup,describingthesymmetriesofthe modularfunctions soimportantinmodernnumbertheory;andthelistgoeson ... ThesecondpairofinnovationswithrespecttothecontentsofVCAiscentred ontheuseof vectorfields asanalternativemeansofvisualizingcomplexmappings. Insteadofpicturingapoint z in C asbeingmappedtoanotherpoint w = f(z) inanothercopyof C—whichistheparadigminforcethroughoutthefirstnine chapters—weinstead picture f(z) asavectoremanatingfrom z
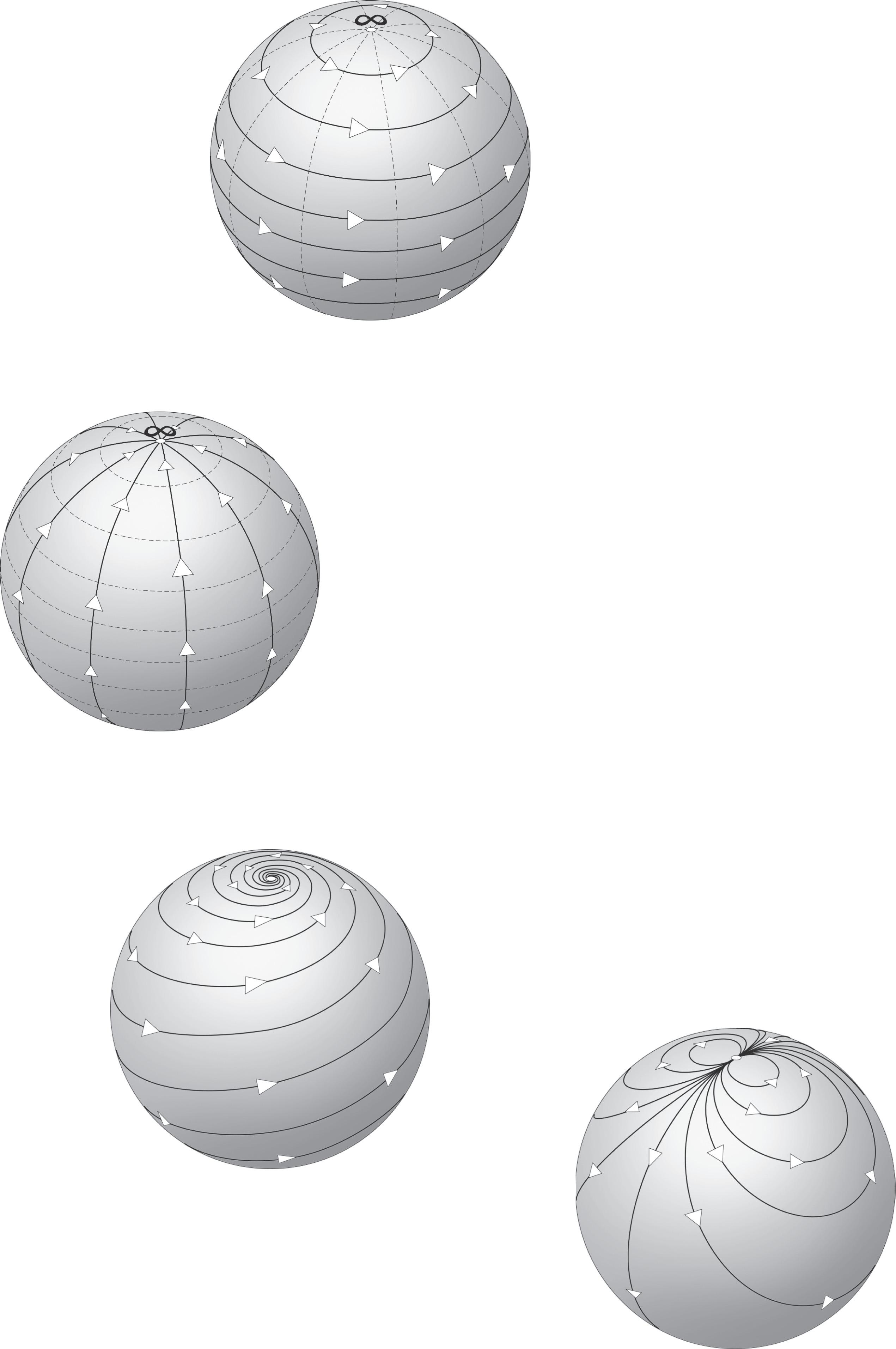
• TheTopologyofVectorFields isthesubjectofChapter10.Hereweshed newlightonthevitaltopologicalconceptofthe windingnumber (thesubject ofChapter7)byinsteadviewingitthroughtheprismofthe index ofasingular pointofavectorfield.Theclimaxofthetopologicalanalysisisaproofofthe gloriousPoincaré–HopfTheorem,(10.4),whichrelatestheindicesofaflowona closedsurfacetothesurface’stopological genus,whichcountshowmanyholes thesurfacehas.
SofarasIcantell,myfinal22 majorinnovativetopicinVCAhas still nothadthe impactIbelieveitdeserves,despitemybestefforts,anddespitetheevenearlier, independenteffortsofitsfirstprincipalchampion,ProfessorBartBraden,who shouldalsobecredited23 withhaving named theconceptinhonourofitsfirst proponent:
• ThePólyaVectorField ofacomplexmapping z ÞÑ f(z) wasintroducedby GeorgePólya(1887–1985)inhis1974textbookwithGordonLatta,(Pólyaand Latta, 1974),anditisthesubjectofVCA’sChapter11:Ateachpoint z in C wedrawavector P(z) thatisdefinedtobethe complexconjugate of f(z),so P(z) ≡ f(z).This Pólyavectorfield thenprovidesawonderfullyvividmeansof visualizing therealandimaginarypartsoftheintegral24 of f(z) alongadirected path(akaa contour) C connectingtwopointsin C,namely,(11.1)onpage 549:
C f(z) dz = [flowof P along C] + i [flowof P across C]
Here,theimaginaryfluxcomponentis positive if P flowsacross C fromourlefttoour right aswefaceforwardinthedirectionoftravelalong C. Next,itfollowsimmediatelyfromthe Cauchy–Riemannequations,(4.7),that thereisawonderfullyvivid physicalinterpretation oftheexistenceofthecomplex derivative(whatIcallthe amplitwist),namely,
Ifandonlyiftheamplitwist f′(z) exists,thenthePólyavector field P(z) ≡ f(z) is divergence-free and curl-free.
Thisinturnimmediatelyprovidesamarvellously physicalexplanation ofonethe centralresultsofComplexAnalysis,namely, Cauchy’sTheorem,(8.20),thesimplest versionofwhichstatesthat
Iftheamplitwist f′ existseverywhereonandinsideasimple closedloop L, then L f(z)dz = 0.
Butif L containsasingularityof f(z),thentheintegralneednotvanish.Anexample ofcentralimportanceis f(z)=(1/z),forwhichthePólyavectorfieldistheradial outwardflowfroma source attheorigin.Choosing L tobeanorigin-centredcircle
22 Infacttherearemanyotherinnovationsscatteredacrossthework,mostnotablymyintroduction ofaconceptIchristenedthe complexcurvature,whichIapplytocentralforcefieldsinChapter5,and tothe geometry ofharmonicfunctionsinChapter12;there’salsothe TopologicalArgumentPrinciple in Chapter7,andmorebesides,butIcannotattempttocatalogue all theseideasanddiscoverieshere. SomeoftheobservationsinChapter12werepreviouslypublishedin Needham (1994),whichwonthe MAA’s CarlB.AllendoerferAward in1995.
23 See Braden (1985), Braden (1987)—whichwontheMAA’s CarlB.AllendoerferAward in1988—and Braden (1991).
24 Restassuredthatthefollowingideaswillallbeexplained abinitio inthemaintext;thissection ofthePrefaceisaddressedtoexperiencedreaderswhoare alreadyfamiliarwith thefundamentalsof standardComplexAnalysis.
K ofradius r,tracedcounterclockwise,weobtainan immediatevisualandphysical explanation oftheiconicfactthat
Forclearlythereisnoflowalong K,andsince P(z)=(1/z) flowsorthogonally across K (fromlefttoright)withspeed (1/r),itsfluxacross K is (2πr)(1/r)= 2π Furthermore,thisphysicalinterpretationexplainswhythevalueoftheintegralwill remain 2πi if K iscontinuouslydeformedintoageneralloopencirclingthesource attheorigin,solongas K doesnot cross thatpointasitisdeformed.
Lastly,theabilityofmoderncomputerstoquicklyandeasilydrawthePólya vectorfieldofanyexplicitformulafor f(z) makestheconceptallthemorepowerful asameansof visualizing ComplexAnalysis.
WhiletheevangelicalworkofVCA(andofBraden’searlierpapers)failedto haveitsdesiredeffectinthe20th century,myferventhopenowisthatbyexplicitlysinglingoutthisconceptforpraiseinthisnewPreface,morepeoplemaytake noticeofit,andthegospelofthePólyavectorfieldmaytherebybespreadamongst multitudesofnewbelieversinthe21st century!
TheQuietRevolution
Inhindsight,somethingwasclearlyintheair.NosoonerhadVCAbeenpublished thanIbegantonoticetheemergenceofawaveofkindredworksthatlikewise challengedtheprevailingdominanceofanarid,purelyformalapproachtomathematics,andthatinsteadembracedintuitiveexplanations,themeaningofresults, and,crucially,therevelatorypowerof geometry
ItappearedthatIhadunwittinglybeenenlistedintoaresistancemovement ofsorts,oneinwhichcellmemberswerenotpermittedtoknoweachothers identities—perhapsfortheirownsafety?!But,unlikeBourbaki,thisquietrevolutionhadnonameandnoleaders;itwas,tocoinaphrase,“Bythepeople,for thepeople.”Longmaythishealthyembraceof meaning,intuition,and geometry continue! Cheers! Iraisemyglasstothe next 25years!
T.N. MillValley,California June,2022
PREFACE
Theoriesoftheknown,whicharedescribedbydifferentphysicalideas,maybeequivalent inalltheirpredictionsandhencescientificallyindistinguishable.However,theyarenot psychologicallyidenticalwhentryingtomovefromthatbaseintotheunknown.Fordifferentviewssuggestdifferentkindsofmodificationswhichmightbemadeandhencearenot equivalentinthehypothesesonegeneratesfromtheminone’sattempttounderstandwhat isnotyetunderstood.
AParable
Imagineasocietyinwhichthecitizensareencouraged,indeedcompelledupto acertainage,toread(andsometimeswrite)musicalscores.Allquiteadmirable. However,thissocietyalsohasaverycurious—fewrememberhowitallstarted— anddisturbinglaw: Musicmustneverbelistenedtoorperformed!
Thoughitsimportanceisuniversallyacknowledged,forsomereasonmusicis notwidelyappreciatedinthissociety.Tobesure,professorsstillexcitedlypore overthegreatworksofBach,Wagner,andtherest,andtheydotheirutmostto communicatetotheirstudentsthebeautifulmeaningofwhattheyfindthere,but theystillbecometongue-tiedwhenbrashlyaskedthequestion,“What’sthepoint ofallthis?!”
Inthisparable,itwaspatentlyunfairandirrationaltohavealawforbiddingwould-bemusicstudentsfromexperiencingandunderstandingthesubject directlythrough“sonicintuition.”Butinoursocietyofmathematicianswe have suchalaw.Itisnotawrittenlaw,andthosewhofloutitmayyetprosper,butit says, Mathematicsmustnotbevisualized!
Morelikelythannot,whenoneopensarandommodernmathematicstextona randomsubject,oneisconfrontedbyabstractsymbolicreasoningthatisdivorced fromone’ssensoryexperienceoftheworld, despite thefactthattheveryphenomenaoneisstudyingwereoftendiscoveredbyappealingtogeometric(andperhaps physical)intuition.
Thisreflectsthefactthatsteadilyoverthelasthundredyearsthehonourof visualreasoninginmathematicshasbeenbesmirched.Althoughthegreatmathematicianshavealwaysbeenoblivioustosuchfashions,itisonlyrecentlythatthe “mathematicianinthestreet”haspickedupthegauntletonbehalfofgeometry.
Feynman (1966)
Thepresentbookopenlychallengesthecurrentdominanceofpurelysymbolic logicalreasoningbyusingnew,visuallyaccessibleargumentstoexplainthetruths ofelementarycomplexanalysis.
Computers
Inpart,theresurgenceofinterestingeometrycanbetracedtothemass-availability ofcomputerstodrawmathematicalobjects,andperhapsalsototherelated,somewhatbreathless,popularinterestinchaostheoryandinfractals.Thisbookinstead advocatesthemoresoberuseofcomputersasanaidtogeometric reasoning
Ihavetriedtoencouragethereadertothinkofthecomputerasaphysicistwould hislaboratory—itmaybeusedtocheckexistingideasabouttheconstructionof theworld,orasatoolfordiscoveringnewphenomenawhichthendemandnew ideasfortheirexplanation.ThroughoutthetextIhavesuggestedsuchusesofthe computer,butIhavedeliberatelyavoidedgiving detailed instructions.Thereason issimple:whereasamathematicalideaisatimelessthing,fewthingsaremore ephemeralthancomputerhardwareandsoftware.
Havingsaidthis,theprogram“f(z)”iscurrently25 thebesttoolforvisually exploringtheideasinthisbook;afreedemonstrationversioncanbedownloaded directlyfromLascauxGraphics.Onoccasionitwouldalsobehelpfulifonehad accesstoanall-purposemathematicalenginesuchas Maple or Mathematica.However,Iwouldliketostressthatnoneoftheabovesoftwareisessential:theentire bookcanbefullyunderstoodwithout any useofacomputer.
Finally,somereadersmaybeinterestedinknowinghowcomputerswereused toproducethisbook.Perhapsfiveofthe501diagramsweredrawnusingoutput from Mathematica;theremainderIdrewbyhand(orrather“bymouse”)using CorelDRAW,occasionallyguidedbyoutputfrom“f(z)”.Itypesetthebookin LATEX usingthewonderful Y&YTEXSystemforWindows26,thefiguresbeingincludedas EPSfiles.ThetextisTimes27,withHelveticaheads,andthemathematicsisprincipallyMathTime,thoughnineothermathematicalfontsmakecameoappearances. AlloftheseAdobeType1fontswereobtainedfromY&Y,Inc.,withtheexception ofAdobe’s MathematicalPi-Six font,whichIusedtorepresentquaternions.Finally, OUPprintedthebookdirectlyfrommyPostScriptfile.
TheBook’sNewtonianGenesis
ItisfairlywellknownthatNewton’soriginal1665versionofthecalculuswasdifferentfromtheonewelearntoday:itsessencewasthemanipulationofpower
25 Sadly,thisnolongerexists.
26 Sadly,thisnolongerexists.
27 Inthisnewedition,theheadingsareOptima,thetextisPalatino,andthemathematicsisEuler.
series,whichNewtonlikenedtothemanipulationofdecimalexpansionsinarithmetic.Thesymboliccalculus—theoneineverystandardtextbook,andtheonenow associatedwiththenameofLeibniz—wasalsoperfectlyfamiliartoNewton,but apparentlyitwasofonlyincidentalinteresttohim.Afterall,armedwithhispower series,Newtoncouldevaluateanintegrallike e x2 dx justaseasilyas sinxdx. LetLeibniztry that!
Itislesswellknownthataround1680Newtonbecamedisenchantedwithboth theseapproaches,whereuponheproceededtodevelopa third versionofcalculus,basedon geometry.This“geometriccalculus”isthemathematicalenginethat propelsthebrilliantphysicsofNewton’s Principia
HavinggraspedNewton’smethod,Iimmediatelytriedmyownhandatusingit tosimplifymyteachingofintroductorycalculus.Anexamplewillhelptoexplain whatImeanbythis.Letusshowthatif T = tan θ,then dT dθ = 1 + T 2.Ifweincrease θ byasmallamount dθ then T willincreasebytheamount dT inthefigurebelow. Toobtaintheresult,weneedonlyobservethatinthelimitas dθ tendstozero,the blacktriangleisultimatelysimilar[exercise]totheshadedtriangle.Thus,inthis limit,
OnlygraduallydidIcometorealizehownaturallythismodeofthoughtcouldbe applied—almostexactly300yearslater!—tothegeometryofthecomplexplane.
ReadingThisBook
Inthehopeofmakingthebookfuntoread,Ihaveattemptedtowriteasthough Iwereexplainingtheideasdirectlytoafriend.Correspondingly,Ihavetried tomakeyou,thereader,intoanactiveparticipantindevelopingtheideas.For example,asanargumentprogresses,Ihavefrequentlyanddeliberatelyplaceda pairoflogicalsteppingstonessufficientlyfarapartthatyoumayneedtopause
andstretchslightlytopassfromonetothenext.Suchplacesaremarked“[exercise]”;theyoftenrequirenothingmorethanasimplecalculationoramomentof reflection.
Thisbringsmetotheexercisesproper,whichmaybefoundattheendofeach chapter.Inthebeliefthattheessentialprerequisiteforfindingtheanswertoa questionisthe desire tofindit,Ihavemadeeveryefforttoprovideexercisesthat provokecuriosity.Theyareconsiderablymorewide-rangingthaniscommon,and theyoftenestablishimportantfactswhicharethenusedfreelyinthetextitself. Whileproblemswhosebeallandendallisroutinecalculationaretherebyavoided, Ibelievethatreaderswillautomaticallydevelopconsiderablecomputationalskill intheprocess ofseekingsolutionstotheseproblems.Ontheotherhand,myintentioninalargenumberoftheexercisesistoillustratehowgeometricthinkingcan often replace lengthycalculation.
Anypartofthebookmarkedwithastar(“*”)maybeomittedonafirstreading.Ifyoudoelecttoreadastarredsection,youmayinturnchoosetoomitany starred subsections.Pleasenote,however,thatapartofthebookthatisstarredis notnecessarilyanymoredifficult,noranylessinterestingorimportant,thanany otherpartofthebook.
TeachingfromthisBook
Theentirebookcanprobablybecoveredinayear,butinasinglesemestercourse onemustfirstdecidewhat kind ofcoursetoteach,thenchooseacorresponding paththroughthebook.HereIofferjustthreesuchpossiblepaths:
• TraditionalCourse. Chapters1to9, omittingallstarredmaterial (e.g.,thewhole ofChapter6).
• VectorFieldCourse. InordertotakeadvantageofthePólyavectorfield approachtovisualizingcomplexintegrals,onecouldfollowthe“TraditionalCourse”above,omittingChapter9,andaddingtheunstarredpartsof Chapters10and11.
• Non-EuclideanCourse. Attheexpenseofteachinganyintegration,onecould giveacoursefocusedonMöbiustransformationsandnon-Euclideangeometry. Thesetworelatedpartsofcomplexanalysisareprobablythemostimportant onesforcontemporarymathematicsandphysics,andyettheyarealsotheones thatarealmostentirelyneglectedinundergraduate-leveltexts.Ontheother hand,graduate-levelworkstendtoassumethatyouhavealreadyencountered themainideasasanundergraduate:Catch22!
Suchacoursemightgoasfollows:AllofChapter1;theunstarredpartsof Chapter2;allofChapter3,includingthestarredsectionsbut(possibly)omitting
thestarred subsections;allofChapter4;allofChapter6,includingthestarred sectionsbut(possibly)omittingthestarred subsections.
OmissionsandApologies
Ifonebelievesintheultimateunityofmathematicsandphysics,asIdo,thena verystrongcaseforthenecessityofcomplexnumberscanbebuiltontheirapparentlyfundamentalroleinthequantummechanicallawsgoverning matter.Also,the workofSirRogerPenrosehasshown(withincreasingforce)thatcomplexnumbers playanequallycentralroleintherelativisticlawsgoverningthestructureof spacetime.Indeed,ifthelawsofmatterandofspacetimeareevertobereconciled,thenit seemsverylikelythatitwillbethroughtheauspicesofthecomplexnumbers.This bookcannotexplorethesematters;instead,werefertheinterestedreaderto Feynman (1963,Vol.III), Feynman (1985), PenroseandRindler (1984),andespecially Penrose (2005).
Amoreseriousomissionisthelackofdiscussionof Riemannsurfaces,whichI hadoriginallyintendedtotreatinafinalchapter.Thisplanwasabortedonceit becameclearthataserioustreatmentwouldentailexpandingthebookbeyond reason.Bythistime,however,Ihadalreadyerectedmuchofthenecessaryscaffolding,andthismaterialremainsinthefinishedbook.Inparticular,Ihopethat theinterestedreaderwillfindthelastthreechaptershelpfulinunderstandingRiemann’soriginalphysicalinsights,asexpoundedby Klein (1881).Seealso Springer (1981,Ch.1),whichessentiallyreproducesKlein’smonograph,butwithadditional helpfulcommentary.
Iconsiderthehistoryofmathematicstobeavitaltoolinunderstandingboththe currentstateofmathematics,anditstrajectoryintothefuture.Sadly,however,Ican donomorethantouchonhistoricalmattersinthepresentwork;insteadIreferyou totheremarkablebook, MathematicsandItsHistory,byJohn Stillwell (2010).Indeed, Istronglyencourageyoutothinkofhisbookasacompaniontomine:notonlydoes ittraceandexplainthedevelopmentofcomplexanalysis,butitalsoexploresand illuminatestheconnectionswithotherareasofmathematics.
TotheexpertreaderIwouldliketoapologizeforhavinginventedtheword “amplitwist”[Chapter4]asasynonym(moreorless)for“derivative”,aswellthe componentterms“amplification”and“twist”.Icanonlysaythattheneedfor some suchterminologywasforcedonmeintheclassroom:ifyoutryteachingtheideas inthisbook without usingsuchlanguage,Ithinkyouwillquicklydiscoverwhat Imean!Incidentally,aprecedenceargumentindefenceof“amplitwist”mightbe thatasimilartermwascoinedbytheolderGermanschoolofKlein,Bieberbach, etal.Theyspokeof“eineDrehstreckung”,from“drehen”(totwist)and“strecken” (tostretch).
Asignificantproportionofthegeometricobservationsandargumentscontained inthisbookare,tothebestofmyknowledge,new.Ihavenotdrawnattentionto thisinthetextitselfasthiswouldhaveservednousefulpurpose:studentsdon’t needtoknow,andexpertswillknowwithoutbeingtold.However,incaseswhere anideaisclearlyunusualbutIamawareofithavingbeenpublishedbysomeone else,Ihavetriedtogivecreditwherecreditisdue.
Inattemptingtorethinksomuchclassicalmathematics,Ihavenodoubtmade mistakes;theblamefortheseisminealone.Correctionswillbegratefullyreceived atVCA.correction@gmail.com.
MybookwillnodoubtbeflawedinmanywaysofwhichIamnotyetaware, butthereisone“sin”thatIhaveintentionallycommitted,andforwhichIshall notrepent:manyoftheargumentsarenotrigorous,atleastastheystand.Thisis aseriouscrimeifonebelievesthatourmathematicaltheoriesaremerelyelaborate mentalconstructs,precariouslyhoistedaloft.Thenrigourbecomesthenerverackingbalancingactthatpreventstheentirestructurefromcrashingdownaround us.Butsupposeonebelieves,asIdo,thatourmathematicaltheoriesareattemptingtocaptureaspectsofarobustPlatonicworldthatisnotofourmaking.Iwould thencontendthataninitiallackofrigourisasmallpricetopayifitallowsthe readertoseeintothisworldmoredirectlyandpleasurablythanwouldotherwise bepossible.
T.N. SanFrancisco,California
June,1996