Topology SecondEdition
LibraryofCongressCataloging-in-PublicationData
Munkres,Jame8R Topology/JamesRaymondMunkres--2nded p cm
Includesbibliographicalreferencesandindex.
ISBN0-13-181629-2
1 Topology I.Title. QA61IP482 2000 514--dc2l
99-052942 CIP
AcquisitionsEditor:GeorgeLobell AssistantVicePresidentofProductionandManufactunngDavidWRiccardi ExecutiveManagingEditorKathleenSchiaparelli SeniorManagingEditor.LrndaMihoIovBehrens ProductionEditorLynnM.Savino ManufactunngBuyer.AlanFischer ManufactunngManager.TrudyPisciotti MarketingManager.MelodyMarcus MarketingAssistantVuweJansen DirectorofMarketingJohn EditonalAssistantGaleEpps ArtDirectorJayneConte CoverDesignerBruceKensdaar CompositionMacroTeXServices
©2000,1975byPrenticeHail,Inc. UpperSaddleRiver,NJ07458
AllngbtsreservedNopartofthisbookmay bereproduced,inanyformorbyanymeans, withoutpermissioninwntingfromthepublisher.
PrintedintheUnitedStatesofAmenca 10987
ISBN0—3.3—3.83.629—2
PRENTICE-HALLINTERNATEONAL(UK)LEMETED,LONDON
PRENTECE-HALLOFAUSTRALEAPrYLEMETED,SYDNEY PRENTICE-HALLCANADA,INC,TORONTO
PRENTECE-HALLHESPANOAMERECANA,SA,MExICO
PRENTECE-HALLOFINDIAPREVATELEMITED,NEWDELHE
PRENTECE-HALLOFJAPAN,INC,ToKYo
PEARSONEDUCATEONASEAPTELTD
EDETORAPRENTICE-HALLDOBRASEL,LTDA,RIODEJANEERO
ForBarbara
Chapter12ClassificationofSurfaces
74FundamentalGroupsofSurfaces
75HomologyofSurfaces
76CuttingandPasting
77TheClassificationTheorem
78ConstructingCompactSurfaces
Chapter13ClassificationofCoveringSpaces
79EquivalenceofCoveringSpaces 80TheUniversalCoveringSpace
CoveringTransformations
82ExistenceofCoveringSpaces ssuppLementaryExercises:TopologicalPropertiesand
Chapter14ApplicationstoGroupTheory.
83CoveringSpacesofaGraph
84TheFundamentalGroupofaGraph
85SubgroupsofFreeGroups
Bibliography Index
Preface
Thisbookisintendedasatextforaone-ortwo-semesterintroductiontotopology,at thesenioror graduatelevel.
ThesubjectoftopoLogyisofinterestinitsownright,anditalsoservestolaythe foundationsforfuturestudyinanalysis,ingeometry,andinalgebraictopology.There isnouniversalagreementamongmathematiciansastowhatafirstcourseintopology shouldinclude;therearemanytopicsthatareappropriatetosuchacourse,andnotall areequallyrelevanttothesedifferingpurposes.Inthechoiceofmaterialtobetreated, Ihavetriedtostrikeabalanceamongthevariouspointsofview.
Prerequisites.Therearenoformalsubjectmatterprerequisitesforstudyingmostof thisbook.Idonotevenassumethereaderknowsmuchsettheory.Havingsaidthat, ImusthastentoaddthatunlessthereaderhasstudiedabitofanaLysisor"rigorous calculus:'muchofthemotivationfortheconceptsintroducedinthefirstpartofthe bookwillbemissing.Thingswillgomoresmoothlyifheorshealreadyhashadsome experiencewithcontinuousfunctions,openandclosedsets,metricspaces.andthe like,althoughnoneoftheseactuallyassumed.InPartII,wedoassumefamiliarity withtheelementsofgrouptheory.
Moststudentsinatopologycoursthave,inmyexperience,someknowledgeof thefoundationsofmathematics.Buttheamountvariesagreatdealfromonestudent toanother.Therefore,Ibeginwithafairlythoroughchapteronsettheoryandlogic.It startsatanelementarylevelandworksuptoalevelthatmightbedescribedas"semisophisticated."Ittreatsthosetopics(andonlythose)thatwillbeneededlaterinthe book.Moststudentswillalreadybefamiliarwiththematerialofthefirstfewsections, butmanyofthemwillfindtheirexpertisedisappearingsomewhereaboutthemiddle
ofthechapter.Howmuchtimeandefforttheinstructor11needtospendonthis chapterwilLthusdependLargelyonthemathematicalsophisticationandexperienceof thestudents.Abilitytodotheexercisesfairlyreadily(andcorrectly!)shouldserveas areasonablecriterionfordeterminingwhetherthestudent'smasteryofsettheoryis sufficientforthestudenttobeginthestudyoftopology.
Manystudents(andinstructors!)wouldprefertoskipthefoundationalmaterial ofChapter1andjumprightintothestudyoftopology.Oneignoresthefoundations, however,onlyattheriskoflaterconfusionanderror.Whatonecandoistotreat initiallyonlythosesectionsthatareneededatonce,postponingtheremainderuntil theyareneeded.Thefirstsevensections(throughcountability)areneededthroughout thebook;Iusuallyassignsomeofthemasreadingandlectureontherest.Sections9 and10,ontheaxiomofchoiceandwell-ordering,arenotneededuntilthediscussion ofcompactnessinChapter3.Section11,onthemaximumprinciple,canbepostponed evenlonger;itisneededonlyfortheTychonofftheorem(Chapter5)andthetheorem onthefundamentalgroupofalineargraph(Chapter14).
Howthebookisorganized.Thisbookcanbeusedforanumberofdifferentcourses. Ihaveattemptedtoorganizeasflexiblyaspossible.soastoenabLetheinstructorto followhisorherownpreferencesinthematter.
PartI,consistingofthefirsteightchapters,isdevotedtothesubjectcommonly calledgeneraltopology.Thefirstfourchaptersdealwiththebodyofmaterialthat, inmyopinion,shouldbeincludedinanyintroductorytopologycourseworthyofthe name.Thismaybeconsideredthe"irreduciblecore"ofthesubject,treatingasitdoes settheory,topologicalspaces,connectedness,compactness(throughcompactnessof finiteproducts),andthecountabilityandseparationaxioms(throughtheUrysohn metrizationtheorem).TheremainingfourchaptersofPartIexploreadditionaltopics; theyareessentiallyindependentofoneanother,dependingononlythecorematerial ofChapters1-4.Theinstructormaytakethemupinanyorderheorshechooses.
PartIIconstitutesanintroductiontothesubjectofAlgebraicTopology.Itdepends ononlythecorematerialofChapters1—4.Thispartofthebooktreatswithsome thoroughnessthenotionsoffundamentalgroupandcoveringspace,alongwiththeir manyandvariedapplications.SomeofthechaptersofPartIIareindependentofone another;thedependenceamongthemisexpressediiithefoLlowingdiagram:
Certainsectionsofthebookaremarkedwithanasterisk;thesesectionsmaybe omittedorpostponedwithnolossofcontinuity.Certaintheoremsaremarkedsimilarly.Anydependenceoflatermaterialontheseasteriskedsectionsortheoremsis indicatedatthetime,andagainwhentheresultsareneeded.Someoftheexercises alsodependonearlierasteriskedmaterial,butinsuchcasesthedependenceisobvious.
Setsofsupplementaryexercisesappearattheendsofseveralofthechapters.They provideanopportunityforexplorationoftopicsthatdivergesomewhatfromthemain thrustofthebook;anambitiousstudentmightuseoneasabasisforanindependent paperorresearchproject.Mostarefairlyself-contained,buttheoneontopological groupshasasasequelanumberofadditionalexercisesonthetopicthatappearinlater sectionsofthebook.
Possiblecourseoutlines.Mostinstructorswhousethistextforacourseingeneral topologywillwishtocoverChapters1—4,alongwiththe theoreminChapter5.Manywillcoveradditionaltopicsaswell.Possibilitiesincludethefollowing: theStone-techcompactification metrizationtheorems(Chapter6),thePeano curve Ascoli'stheorem and/or§47),anddimensiontheory Ihave, indifferentsemesters,followedeachoftheseoptions.
Foraone-semestercourseinalgebraictopology,onecanexpecttocovermostof PartH.
Itisalsopossibletotreatbothaspectsoftopologyinasinglesemester,although withsomecorrespondinglossofdepth.Onefeasibleoutlineforsuchacoursewould consistofChapters1—3,followedbyChapter9;thelatterdoesnotdependonthe materialofChapter4.(Thenon-asteriskedsectionsofChapters10and13alsoare independentofChapter4.)
Commentsonthisedition.Thereaderwhoisfamiliarwiththefirsteditionofthis bookwillfindnosubstantialchangesinthepartofthebookdealingwithgeneral topology.Ihaveconfinedmyselflargelyto"fine-tuning"thetextmaterialandthe exercises.However,thefinalchapterofthefirstedition,whichdealtwithalgebraic topology,hasbeensubstantiallyexpandedandrewritten.IthasbecomePartIIofthis book.Intheyearssincethefirsteditionappeared,ithasbecomeincreasinglycommon tooffertopologyasatwo-termcourse,thefirstdevotedtogeneraltopologyandthe secondtoalgebraictopology.Byexpandingthetreatmentofthelattersubject,Ihave intendedtomakethisrevisionservetheneedsofsuchacourse.
Acknowledgments.MostofthetopologistswithwhomIhavestudied,orwhose booksIhaveread,havecontributedinonewayoranothertothisbook;Imention onlyEdwinMoise,RaymondWilder,GailYoung,andRaoulBott,buttherearemany others.Fortheirhelpfulcommentsconcerningthisbook,mythankstoKenBrown, RussMcMillan,RobertMosher,andJohnHemperly,andtomycolleaguesGeorge WhiteheadandKennethHoffman.
ThetreatmentofalgebraictopologyhasbeensubstantiallyinfluencedbytheexcellentbookbyWilliamMasseyEM],towhomIexpressappreciation.Finally,thanksare
dueAdamLewenbergofMacroTeXforhisextraordinaryskillandpatienceinsetting textandjugglingfigures.
ButmostofaLl,tomystudentsgomymostheartfeltthanks.FromthemIlearned atleastasmuchastheydidfromme;withoutthemthisbookwouldbeverydifferent.
JR.M.
§1FundamentalConcepts
Hereweintroducetheideasofsettheory,andestablishthebasicterminologyand notation.Wealsodiscusssomepointsofelementarylogicthat,inourexperience,are apttocauseconfusion.
BasicNotation
CommonlyweshallusecapitallettersA,B,...todenotesets,andlowercaseletters a,b,...todenotetheobjectsorelementsbelongingtothesesets.Ifanobjecta belongstoasetA,weexpressthisfactbythenotation
IfadoesnotbelongtoA,weexpressthisfactbywriting
Theequalitysymbol=isusedthroughoutthisbooktomeanlogicalidentity.Thus, whenwewritea=b,wemeanthat"a"and"b"aresymbolsforthesameobject.This iswhatonemeansinarithmetic,forexample,whenonewrites=Similarly,the equationA=Bstatesthat"A"and"B"aresymboLsforthesameset;thatis,AandB consistofpreciselythesameobjects.
Ifaandbaredifferentobjects,wewriteab;andifAandBaredifferentsets, wewriteAB.Forexample,ifAisthesetofallnonnegativerealnumbers,andB isthesetofallpositiverealnumbers,thenAB,becausethenumber0belongstoA andnottoB.
WesaythatAisasubsetofBifeveryelementofAisalsoanelementofB;and weexpressthisfactbywriting ACB.
NothinginthisdefinitionrequiresAtobedifferentfromB;infact,ifA=B,itistrue thatbothAcBandBcA.IfACBandAisdifferentfromB,wesaythatAisa propersubsetofB,andwewrite
AçB.
Therelationscandçarecalledinclusionandproperinclusion,respectively.If ACB,wealsowriteBA,whichisread"BcontainsA."
Howdoesonegoaboutspecifyingaset?Ifthesethasonlyafewelements,one cansimplylisttheobjectsintheset,writing"Aisthesetconsistingoftheelementsa, b,andc."Insymbols,thisstatementbecomes A=(a,b,c), wherebracesareusedtoenclosethelistofeLements.
Theusualwaytospecifyaset,however,istotakesomesetAofobjectsandsome propertythatelementsofAmayormaynotpossess,andtoformthesetconsistfng ofallelementsofAhavingthatproperty.Forinstance,onemighttakethesetof realnumbersandformthesubsetBconsistingofallevenintegers.Insymbols,this statementbecomes
B={xxisaneveninteger).
Herethebracesstandforthewords"thesetof,"andtheverticalbarstandsforthe words"suchthat."Theequationisread"Bisthesetofallxsuchthatxaneven integer."
TheUnionofSetsandtheMeaningof"or"
GiventwosetsAandB,onecanformasetfromthemthatconsistsofalltheelements ofAtogetherwithalltheelementsofB.ThissetiscalledtheunionofAandBand isdenotedbyAUB.Formally,wedefine
AUB={xIxEAorxEB).
Butwemustpauseatthispointandmakesureexactlywhatwemeanbythestatement "xEAorxEB."
InordinaryeverydayEnglish,theword"or"isambiguous.Sometimesthestatement"PorQ"means"PorQ,orboth"andsometimesitmeans"PorQ,butnot both."Usuallyonedecidesfromthecontextwhichmeaningisintended.Forexample, supposeIspoketotwostudentsasfollows:
"MissSmith,everystudentregisteredforthiscoursehastakeneitheracoursein linearalgebraoracoursernanalysis."
"Mr.Jones,eitheryougetagradeofatleast70onthefinalexamoryouwillflunk thiscourse"
Inthecontext,MissSmithknowsperfectlywellthatImean"everyonehashadlinear algebraoranalysis,orboth:'andMr.JonesknowsImean"eitherhegetsatleast70 orheflunks,butnotboth."Indeed,Mr.JoneswouLdbeexceedinglyunhappyifboth statementsturnedouttobetrue!
Inmathematics,onecannottoleratesuchambiguity.Onehastopickjustone meaningandstickwithit,orconfusionwillreign.Accordingly,mathematicianshave agreedthattheywillusetheword"or"inthefirstsense,sothatthestatement"PorQ" alwaysmeans"PorQ,orboth."Ifonemeans"PorQ,butnotboth,"thenonehasto includethephrase"butnotboth"explicitly.
Withthisunderstanding,theequationdefiningAUBisunambiguous;itstatesthat AUBisthesetconsistingofallelementsxthatbelongtoAortoBortoboth.
TheIntersectionofSets,theEmptySet,andtheMeaningof"If...Then"
GivensetsAandB,anotherwayonecanformasetistotakethecommonpartofA andB.ThissetiscalledtheintersectionofAandBandisdenotedbyAflB.Formally, wedefine
AflB—{xxEAandxEB).
ButjustaswiththedefinitionofAUB,thereisadifficulty.Thedifficultyisnotinthe meaningoftheword"and";itisofadifferentsort.ItariseswhenthesetsAandB happentohavenoelementsincommon.WhatmeaningdoesthesymbolAflBhave insuchacase?
Totakecareofthiseventuality,wemakeaspecialconvention.Weintroducea specialsetthatwecalltheemptyset,denotedby0,whichwethinkofas"theset havingnoelements."
Usingthisconvention,weexpressthestatementthatAandBhavenoelementsin commonbytheequation
AflB=e.
WealsoexpressthisfactbysayingthatAandBaredisjoint.
Nowsomestudentsarebotheredbythenotionofan"emptyset.""How,"theysay, "canyouhaveasetwithnothinginit?"Theproblemissimilartothatwhicharose manyyearsagowhenthenumber0wasfirstintroduced.
Theemptysetisonlyaconvention,andmathematicscouldverywellgetalong withoutit.Butitisaveryconvenientconvention,foritsavesusagooddealof awkwardnessinstatingtheoremsandinprovingthem.Withoutthisconvention,for instance,onewouldhavetoprovethatthetwosetsAandBdohaveelementsin commonbeforeonecouldusethenotationAflB.Similarly,thenotation
C={xIxEAandxhasacertainproperty)
couldnotbeusedifithappenedthatnoelementxofAhadthegivenproperty.Itis muchmoreconvenienttoagreethatAflBandCequaltheemptysetinsuchcases.
Sincetheemptyset0ismerelyaconvention,wemustmakeconventionsrelating ittotheconceptsalreadyintroduced.Because0isthoughtofas"thesetwithno elements,"itisclearweshouldmaketheconventionthatforeachobjectx,therelation xE0doesnothold.Similarly,thedefinitionsofunionandintersectionshowthatfor everysetAweshouldhavetheequations
AUO=A andAflO=0.
Theinclusionrelationisabitmoretricky.GivenasetA,shouldweagreethat 0CA?Oncemore,wemustbecarefulaboutthewaymathematiciansusetheEnglish language.Theexpression0CAisashorthandwayofwritingthesentence,"Every elementthatbelongstotheemptysetalsobelongstothesetA."Ortoputitmore
formally,"Foreveryobjectx,ifxbelongstotheemptyset,thenxalsobelongstothe setA."
Isthisstatementtrueornot?Somemightsay"yes"andotherssay'no."You willneversettlethequestionbyargument,onlybyagreement.Thisisastatementof theform"IfP,thenQ,"andineverydayEnglishthemeaningofthe"if...then' constructionisambiguous.ItalwaysmeansthatifPistrue,thenQistruealso. Sometimesthatisallitmeans;othertimesitmeanssomethingmore:thatifPisfalse, Qmustbefalse.Usuallyonedecidesfromthecontextwhichinterpretationiscorrect.
Thesituationissimilartotheambiguityintheuseoftheword"or."OnecanreformulatetheexamplesinvolvingMissSmithandMr.Jonestoillustratetheanibiguity. SupposeIsaidthefollowing:
"MissSmith,ifanystudentregisteredforthiscoursehasnottakenacoursein linearalgebra.thenhehastakenacourseinanalysis."
"Mr.Jones,ifyougetagradebelow70onthefinal,youaregoingtoflunkthis course."
Inthecontext,MissSmithunderstandsthatifastudentinthecoursehasnothadlinear algebra,thenhehastakenanalysis,butifhehashadlinearalgebra,hemayormaynot havetakenanalysisaswell.AndMr.Jonesknowsthatifhegetsagradebelow70,he willflunkthecourse,buthegetsagradeofatleast70,hewillpass.
Again,mathematicscannottolerateambiguity,soachoiceofmeaningsmustbe made.Mathematicianshaveagreedalwaystouse"if...then"inthefirstsense,so thatastatementoftheform"IfP.thenQ"meansthatifPistrue,Qistruealso,but ifPisfalse,Qmaybeeithertrueorfalse.
Asanexample,considerthefollowingstatementaboutrealnumbers:
Ifx>0.thenx30.
Itisastatementoftheform,"IfP,thenQ,"wherePisthephrase"x>0"(called thehypothesisofthestatement)andQisthephrase"x30"(calledtheconclusion ofthestatement).Thisisatruestatement,forineverycaseforwhichthehypothesis x>0holds,theconclusionx30holdsaswell.
Anothertruestatementaboutrealnumbersisthefollowing:
Ifx2<0,thenx=23;
ineverycaseforwhichthehypothesisholds,theconclusionholdsaswell.Ofcourse, ithappensinthisexamplethattherearenocasesforwhichthehypothesisholds.A statementofthissortissometimessaidtobevacuouslytrue.
Toreturnnowtotheemptysetandinclusion,weseethattheinclusion0CA doesholdforeverysetA.Writing0CAisthesameassaying,"IfxE0,then xEA?'andthisstatementisvacuouslytrue.
ContrapositiveandConverse
Ourdiscussionofthe"if...then"constructionleadsustoconsideranotherpointof elementarylogicthatsometimescausesdifficulty.Itconcernstherelationbetweena statement,itscontrapositive,anditsconverse.
Givenastatementoftheform"IfP,thenQ,"itscontrapositiveisdefinedtobe thestatement"IfQisnottrue,thenPisnottrue."Forexample,thecontrapositiveof thestatement
ifx>0,thenx30, isthestatement
Ifx3=0,thenitisnottruethatx>0.
Notethatboththestatementanditscontrapositivearetrue.Similarly,thestatement
Ifx2<0.thenx=23, hasasitscontrapositivethestatement
Ifx23,thenitisnottruethatx2<0.
Again,botharetruestatementsaboutrealnumbers.
Theseexamplesmaymakeyoususpectthatthereissomerelationbetweenastatementanditscontrapositive.Andindeedthereis;theyaretwowaysofsayingprecisely thesamething.Eachistrueifandonlyiftheotheristrue;theyarelogically lent.
Thisfactisnothardtodemonstrate.Letusintroducesomenotationfirst.Asa shorthandforthestatement"IfP,thenQ'wewrite
whichisread"PimpliesQ."Thecontrapositivecanthenbeexpressedintheform (notQ) (notP), where"notQ"standsforthephrase"Qisnottrue."
Nowtheonlywayinwhichthestatement"PQ"canfailtobecorrectisifthe hypothesisPistrueandtheconclusionQisfalse.Otherwiseitiscorrect.Similarly, theonlywayinwhichthestatement(notQ) (notP)canfailtobecorrectisif thehypothesis"notQ"istrueandtheconclusion"notP"isfalse.Thisisthesame assayingthatQisfalseandPistrue.Andthis,inturn,ispreciselythesituationin whichPQfailstobecorrect.Thus,weseethatthetwostatementsareeitherboth correctorbothincorrect;theyarelogicallyequivalent.Therefore,weshaLlaccepta proofofthestatement"notQ notP"asaproofofthestatement"P Q."
ThereisanotherstatementthatcanbeformedfromthestatementPQ.Itis thestatement
whichiscalledtheconverseofP Q.Onemustbecarefultodistinguishbetweena statement'sconverseanditscontrapositive.Whereasastatementanditscontrapositive arelogicallyequivalent,thetruthofastatementsaysnothingatallaboutthetruthor falsityofitsconverse.Forexample,thetruestatement
Ifx>0,thenx30,
hasasitsconversethestatement
Ifx30,thenx>0, whichisfalse.Similarly,thetruestatement
Ifx2<0,thenx=23, hasasitsconversethestatement
Ifx=23,then whichisfalse.
IfitshouldhappenthatboththestatementP QanditsconverseQ Pare true,weexpressthisfactbythenotation
P
Q, whichisread"PholdsifandonlyifQholds."
Negation
IfonewishestoformthecontrapositiveofthestatementP Q,onehastoknow howtoformthestatement"notP,"whichiscalledthenegationofP.Inmanycases, thiscausesnodifficulty;butsometimesconfusionoccurswithstatementsinvolvingthe phrases"forevery"and"foratleastone."Thesephrasesarecalledlogicalquantifiers.
Toillustrate,supposethatXisaset,AisasubsetofX,andPisastatementabout thegeneralelementofX.Considerthefollowingstatement:
(*) ForeveryxEA,statementPholds.
Howdoesoneformthenegationofthisstatement?Letustranslatetheprobleminto thelanguageofsets.SupposethatweletBdenotethesetofallthoseelementsx ofXforwhichPholds.Thenstatement(*)isjustthestatementthatAisasubset ofB.Whatisitsnegation?Obviously,thestatementthatAisnotasubsetofB;that is,thestatementthatthereexistsatleastoneelementofAthatdoesnotbelongtoB. Translatingbackintoordinarylanguage,thisbecomes
ForatleastonexEA,statementPdoesnothold
Therefore,toformthenegationofstatement(*),onereplacesthequantifier"forevery" bythequantifier"foratleastone,"andonereplacesstatementPbyitsnegation.
ForatleastonexEA,statementQholds,
Theprocessworksinreversejustaswell;thenegationofthestatement isthestatement
ForeveryxEA,statementQdoesnothold.
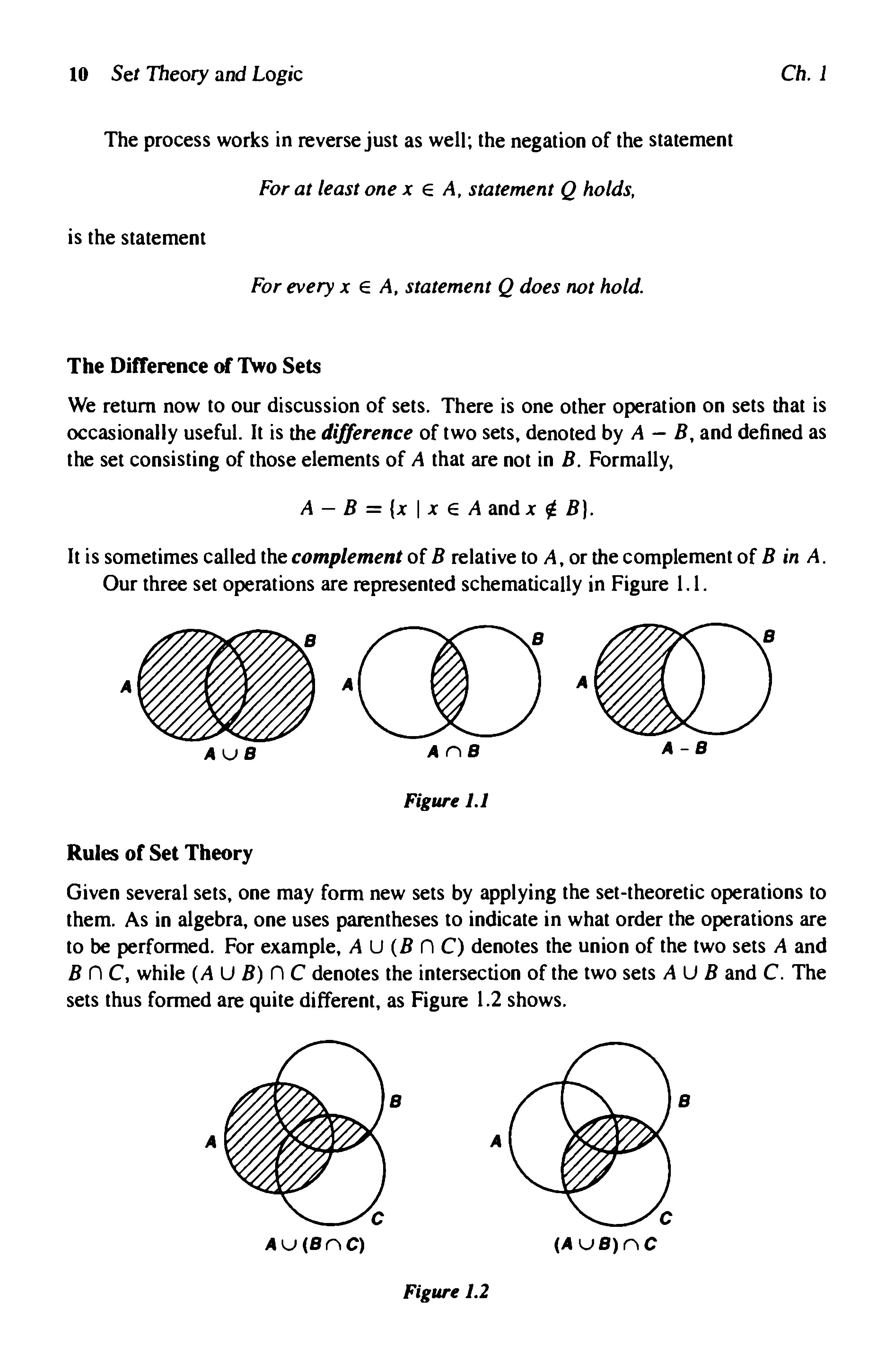
TheDifferenceofTwoSets
Wereturnnowtoourdiscussionofsets.Thereisoneotheroperationonsetsthatis occasionallyuseful.Itisthedifferenceoftwosets,denotedbyA—B,anddefinedas thesetconsistingofthoseelementsofAthatarenotinB.Formally,
ItissometimescalledthecomplementofBrelativetoA,orthecomplementofBinA. OurthreesetoperationsarerepresentedschematicallyinFigure1.1. Al
RulesofSetTheory
Givenseveralsets,onemayformnewsetsbyapplyingtheset-theoreticoperationsto them.Asinalgebra,oneusesparenthesestoindicateinwhatordertheoperationsare tobeperformed.Forexample,AU(BflC)denotestheunionofthetwosetsAand BflC,while(AUB)flCdenotestheintersectionofthetwosetsAUBandC.The setsthusformedarequitedifferent,asFigure1.2shows.
Figure1.2 B
Figure1.1
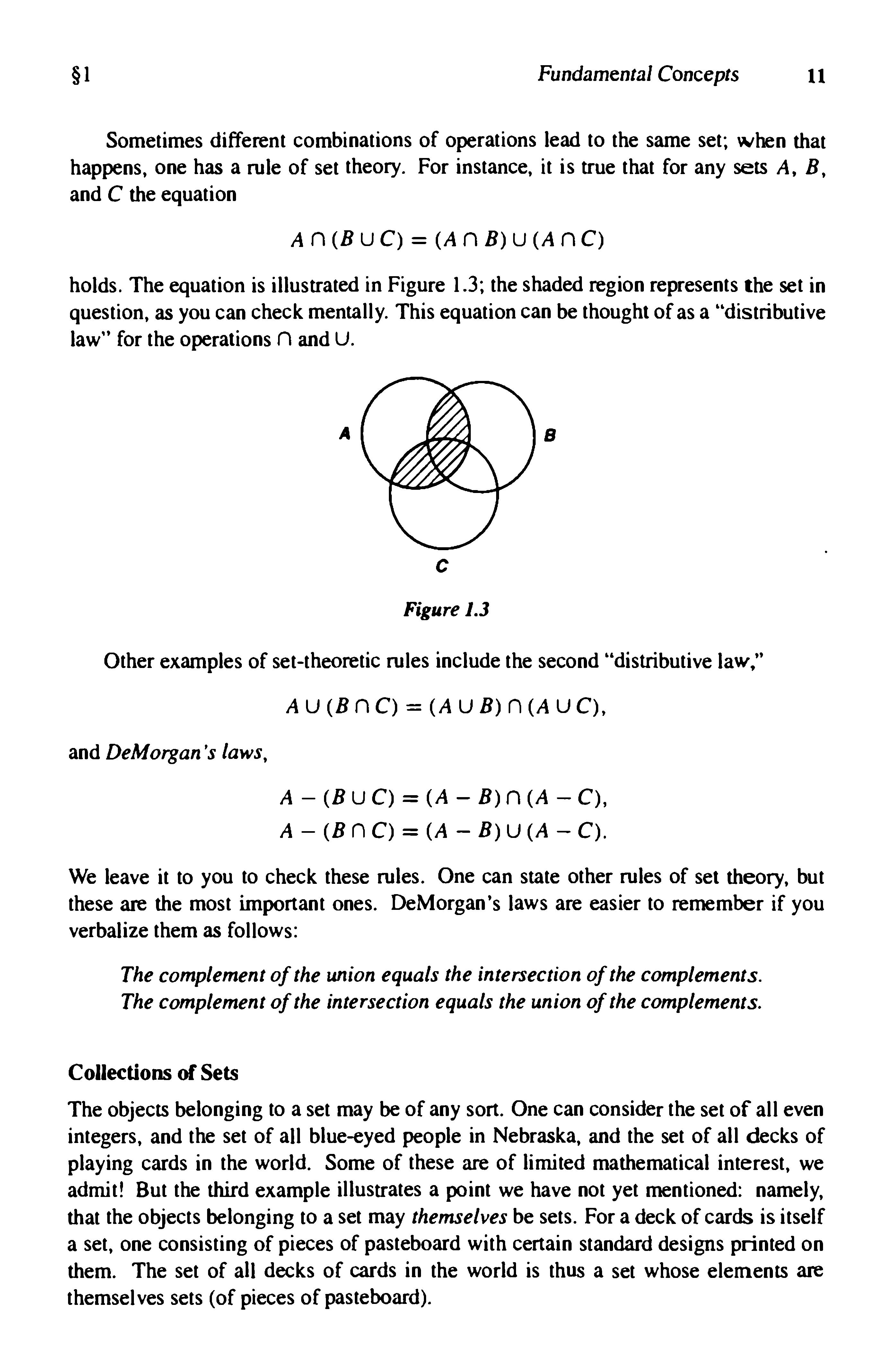
Sometimesdifferentcombinationsofoperationsleadtothesameset;whenthat happens,onehasaruleofsettheory.Forinstance,itistruethatforanysetsA,B, andCtheequation
Afl(BUC)=(AflB)U(AflC)
holds.TheequationisillustratedinFigure1.3;theshadedregionrepresentsthesetin question,asyoucancheckmentally.Thisequationcanbethoughtofasa"distributive law"fortheoperationsflandU.
Otherexamplesofset-theoreticrulesincludethesecond"distributivelaw,"
AU(BflC)=(AUB)fl(AUC), andDeMorgan'slaws,
A—(BUC)=(A—B)fl(A—C), A—(BflC)=(A—B)U(A—C).
Weleaveittoyoutochecktheserules.Onecanstateotherrulesofsettheory,but thesearethemostimportantones.DeMorgan'slawsareeasiertorememberifyou verbalizethemasfollows:
Thecomplementoftheunionequalstheintersectionofthecomplements. Thecomplementoftheintersectionequalstheunionofthecomplements.
CollectionsofSets
Theobjectsbelongingtoasetmaybeofanysort.Onecanconsiderthesetofalleven integers,andthesetofallblue-eyedpeopleinNebraska,andthesetofalldecksof playingcardsintheworld.Someoftheseareoflimitedmathematicalinterest,we admit!Butthethirdexampleillustratesapointwehavenotyetmentioned:namely, thattheobjectsbelongingtoasetmaythemselvesbesets.Foradeckofcardsisitself aset,oneconsistingofpiecesofpasteboardwithcertainstandarddesignsprintedon them.Thesetofalldecksofcardsintheworldisthusasetwhoseelementsare themselvessets(ofpiecesofpasteboard).
Figure1.3
Wenowhaveanotherwaytoformnewsetsfromoldones.GivenasetA,wecan considersetswhoseelementsaresubsetsofA.Inparticular,wecanconsidertheset ofallsubsetsofA.Thissetissometimesdenotedbythesymbol andiscalled thepowersetofA(forreasonstobeexplainedlater).
Whenwehaveasetwhoseelementsaresets,weshalloftenrefertoitasacollectionofsetsanddenoteitbyascriptlettersuchasAor£.Thisdevicewillhelpus inkeepingthingsstraightinargumentswherewehavetoconsiderobjects,andsetsof objects,andcollectionsofsetsofobjects,allatthesametime.Forexample,wemight useAtodenotethecollectionofalldecksofcardsintheworld,lettinganordinary capitalletterAdenoteadeckofcardsandalowercaseletteradenoteasingleplaying card.
Acertainamountofcarewithnotationisneededatthispoint.Wemakeadistinctionbetweentheobjecta,whichisanelementofasetA,andtheone-elementset{a}, whichisasubsetofA.Toillustrate,ifAistheset{a,b,c),thenthestatements
andIntersections
Wehavealreadydefinedwhatwemeanbytheunionandtheintersectionoftwosets. Thereisnoreasontolimitourselvestojusttwosets,forwecanjustaswellformthe unionandintersectionofarbitrarilymanysets.
GivenacollectionAofsets,theunionoftheelementsofAisdefinedbythe equation
A={xxEAforatleastoneAEA).
TheintersectionoftheelementsofAisdefinedbytheequation
ThereisnoproblemwiththesedefinitionsifoneoftheelementsofAhappenstobe theemptyset.Butitisabittrickytodecidewhat(ifanything)thesedefinitionsmean ifweallowAtobetheemptycollection.Applyingthedefinitionsliterally,weseethat noelementxsatisfiesthedefiningpropertyfortheunionoftheelementsofA.Soitis reasonabletosaythat
ifAisemptyOntheotherhand,everyxsatisfies(vacuously)thedefiningpropertyfor theintersectionoftheelementsofA.Thequestionis,everyxinwhatset?Ifonehasa givenlargesetXthatisspecifIedattheoutsetofthediscussiontobeone's"universeof discourse,"andoneconsidersonlysubsetsofXthroughout,itisreasonabletolet
whenAisemptyNotallmathematiciansfollowthisconvention,however.Toavoid difficulty,weshallnordefinetheintersectionwhenAisempty.
CartesianProducts
Thereisyetanotherwayofformingnewsetsfromoldones;itinvolvesthenotionofan "orderedpair"ofobjects.Whenyoustudiedanalyticgeornetiy,thefirstthingyoudid wastoconvinceyourselfthatafteronehaschosenanx-axisanday-axisintheplane, everypointintheplanecanbemadetocorrespondtoauniqueorderedpair(x,y)of realnumbers.(Inamoresophisticatedtreatmentofgeometry,theplaneismorelikely tobedefinedasthesetofallorderedpairsofrealnumbers!)
Thenotionoforderedpaircarriesovertogeneralsets.GivensetsAandB,we definetheircartesianproductAxBtobethesetofallorderedpairs(a,b)forwhicha isanelementofAandbisanelementofB.Formally,
Thisdefinitionassumesthattheconceptof"orderedpair"isalreadygiven.Itcanbe takenasaprimitiveconcept,aswasthenotionof"set';oritcanbegivenadefinitionin termsofthesetoperationsalreadyintroduced.Onedefinitionintermsofsetoperationsis expressedbytheequation
(a,b)=ffaj,
fa,bJJ;
itdefinestheorderedpair(a,b)asacollectionofsets.Ifab,thisdefinitionsaysthat (a,b)isacollectioncontainingtwosets,oneofwhichisaone-elementsetandtheother atwo-elementset.Thefirstcoordinateoftheorderedpairisdefinedtobetheelement belongingtobothsets,andthesecondcoordinateistheelementbelongingtoonlyoneof thesets.Ifa=b,then(a,b)isacollectioncontainingonlyonesetfa},since(a,bJ= a,aJ=(aJinthiscase.Itsfirstcoordinateandsecondcoordinatebothequaltheelement inthissingleset.
Ithinkitisfairtosaythatmostmathematiciansthinkofanorderedpairasapnmitive conceptratherthanthinkingofitasacollectionofsets!
Letusmakeacommentonnotation.Itisanunfortunatefactthatthenotation(a,b) isfirmlyestablishedinmathematicswithtwoentirelydifferentmeanings.Onemeaning,asanorderedpairofobjects,wehavejustdiscussed.Theothermeaningisthe oneyouarefamiliarwithfromanalysis;ifaandbarerealnumbers,thesymbol(a,b) isusedtodenotetheintervalconsistingofallnumbersxsuchthata<x<b.Mostof thetime,thisconflictinnotationwillcausenodifficultybecausethemeaningwillbe clearfromthecontext.Wheneverasituationoccurswhereconfusionispossible,we shalladoptadifferentnotationfortheorderedpair(a,b),denotingitbythesymbol
instead.