Solutions Manual
Solutions to Chapter 1 Problems
The principal stresses are given directly by Eqs (1.11) and (1.12) in which
(or vice versa) and
The directions of the principal stresses are defined by the angle θ in Fig. 1.8(b) in which θ is given by Eq. (1.10). Hence
which gives
It is clear from the derivation of Eqs (1.11) and (1.12) that the first value of θ corresponds to σ I while the second value corresponds to σ II
Finally, the maximum shear stress is obtained from either of Eqs (1.14) or (1.15). Hence from Eq. (1.15)
and will act on planes at 45° to the principal planes.
S.1.1
σ x = 80N/mm2,
y =
τ xy = 45N/mm2. Thus, from Eq. (1.11) 22 I 801 80445 22 σ =++× i.e. σ I = 100.2 N/mm From Eq. (1.12) 2 22 II 801 80445 22 σ =−+× i.e. σ II = – 20.2 N/mm
σ
0
2 245 tan2 1.125 800 θ × ==
θ =
′ and θ =
24°11
114°11′
2 max 100.2(20.2) 60.2N/mm 2 τ = =
The principal stresses are given directly by Eqs (1.11) and (1.12) in which
50N/mm2, σ y = –35 N/mm2 and τ xy = 40 N/mm2. Thus, from Eq. (1.11)
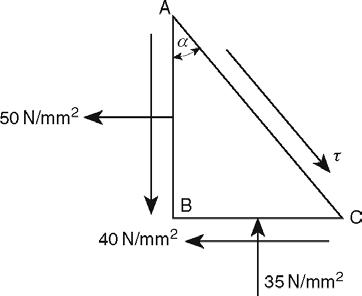
)
Fig. S.1.2
4 Solutions Manual S.1.2
σ x =
22 I 50351 (5035)440 22 σ =+++× i.e. σ I = 65.9 N/mm and from Eq. (1.12) 2 22 II 50351 (5035)440 22 σ =−++× i.e. σ II = –50.9 N/mm From Fig. 1.8(b) and Eq. (1.10) 2 240 tan2 0.941 5035 θ × == +
θ =
′(σ I
θ
II
which gives
21°38
) and
= 111°38 ′(σ
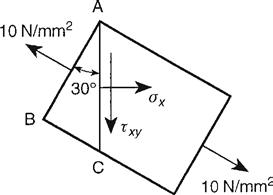
The planes on which there is no direct stress may be found by considering the triangular element of unit thickness shown in Fig. S.1.2 where the plane AC represents the plane on which there is no direct stress. For equilibrium of the element in a direction perpendicular to AC 050ABcos35BCsin40ABsin+40BCcos =−+αααα (i)
Dividing through Eq. (i) by AB
050cos35tansin40sin40tancos =−++αααααα
which, dividing through by cos α, simplifies to
0 = 50–35 tan2
α + 80 tan α
from which
tan α = 2 797 or –0 511
Hence
S.1.3
α = 70°21′ or –27°5′
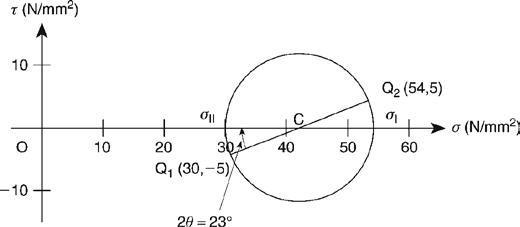
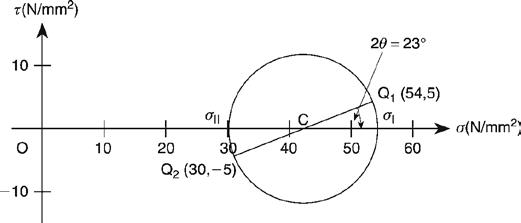
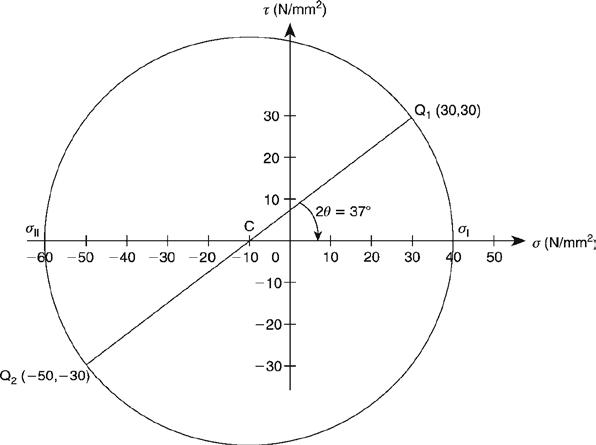
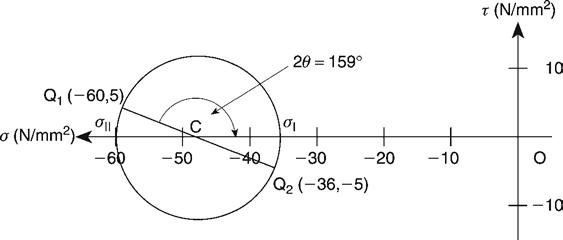
The construction of Mohr’s circle for each stress combination follows the procedure described in Section 1.8 and is shown in Figs S.1.3(a)–(d).
Solutions to Chapter 1 Problems 5
Fig. S.1.3(a)
Fig. S.1.3(b)
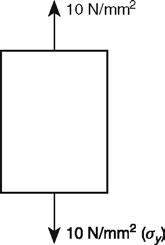
The principal stresses at the point are determined, as indicated in the question, by transforming each state of stress into a σ x , σ y , τ xy stress system. Clearly, in the first case σ x = 0, σ y = 10 N/mm2, τ xy = 0 (Fig. S.1.4(a)). The two remaining cases are transformed by considering the equilibrium of the triangular element ABC in Figs S.1.4(b), (c), (e) and (f). Thus, using the method described in Section 1.6 and the principle of superposition (see Section 5.9), the second stress system of
6 Solutions Manual
Fig. S.1.3(c)
Fig. S.1.3(d) S.1.4
Figs S.1.4(b) and (c) becomes the σ x , σ y , τ xy system shown in Fig. S.1.4(d) while
Solutions to Chapter 1 Problems 7
the third stress system of Figs S.1.4(e) and



into the
system of
8 Solutions Manual
Fig. S.1.4(a)
Fig. S.1.4(b)
Fig. S.1.4(c)
Fig. S.1.4(d)
(f) transforms
σ x , σ y , τ xy
Fig. S.1.4(g).
Finally, the states of stress shown in Figs S.1.4(a), (d) and (g) are superimposed to give the state of stress shown in Fig. S.1.4(h) from which it can be seen that σ
=
II =15N/mm2 and that the x and y planes are principal planes.
Solutions to Chapter 1 Problems 9
I
σ
The geometry of Mohr’s circle of stress is shown in Fig. S.1.5 in which the circle is constructed using the method described in Section 1.8.

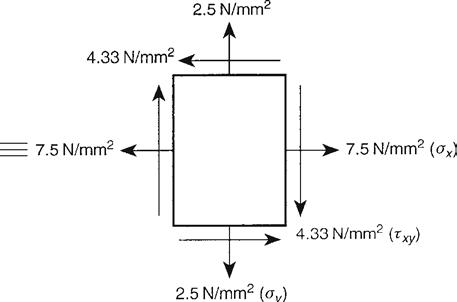
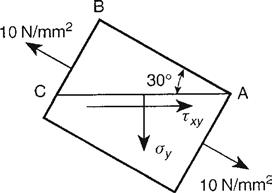
From Fig. S.1.5
10 Solutions Manual
Fig. S.1.4( e )
Fig. S.1.4( f )
Fig. S.1.4(g)
Fig . S.1.4(h)
S.1.5
σ x =
1
1 (i)
OP
= OB– BC +CP
S.1.6
From bending theory the direct stress due to bending on the upper surface of the shaft at a point in the vertical plane of symmetry is given by
From the theory of the torsion of circular section shafts the shear stress at the same point is

Solutions to Chapter 1 Problems 11 Fig. S.1. 5 In Eq. (i) OB = σ I , BC is the radius of the circle which is equal to τ max 2222CPCQQP1111max . xy=−=−ττ and Hence 22 1maxmax xxy σστττ =−+− Similarly 2 221 OPOBBCCPinwhichCPCP y σ ==−− = Thus 22 Imaxmax yxy σστττ =−−−
6 2 4 251075 75N/mm 150/64 x My I σ π ×× == = ×
6 2 4 501075 75N/mm 150/32 xy Tr J τ π ×× == = ×
12 Solutions Manual
Substituting these values in Eqs (1.11) and (1.12) in turn and noting that
The corresponding directions as defined by θ in Fig. 1.8(b)
The direct strains are expressed in terms of the stresses using Eqs (1.42),
σ y = 0 22 I 751 75475 22 σ =++× i.e. 2 I 121.4N/mm σ = 22 II 751 75475 22 σ =−+× i.e. 2 II 46.4N/mm σ =−
(1.10) i.e. 275 tan2 2 750 θ × == Hence I 3143()θσ ′ =° and II 12143()θσ ′ =° S.1.7
are given by Eq.
i.e. 1 [()]xxyz v E εσσσ =−+ (i) 1 [()]yyxz v E εσσσ =−+ (ii) 1 [()]zzxy v E εσσσ =−+ (iii) Then 1 [ 2( )] xyzxyzxyzev E εεεσσσσσσ =++=++−++ i.e. (12) () xyz v e E =++σσσ whence (12) yzx Ee v σσσ +=−
The implication in this problem is that the condition of plane strain also describes the condition of plane stress. Hence, from Eqs (1.52)
Solutions to Chapter 1 Problems 13 Substituting in Eq. (i) 1 12 xxx Ee v Ev εσσ =−− so that (1) 12 xx vEe Ev v εσ=+− Thus (12)(1)(1) xx vEeE vvv σε = + −++ or, since G = E/2(1 + ν) (see Section 1.15) 2 xx eG σλε =+ Similarly 2 yy eG σλε =+ and 2 zz eG σλε =+ S.1.8
1 ()xxy v E εσσ =− (i) 1 ()yyx v E εσσ =− (ii) 2(1) (seeSection1.15) xy xyxy v GE τ γτ + == (iii) The compatibility condition for plane strain is 22 2 22 (seeSection1.11) xyyx xyxy γε ε ∂∂ ∂ =+ ∂∂ ∂∂ (iv) Substituting in Eq. (iv) for ε x , ε y and γ xy from Eqs (i)–(iii), respectively, gives 2 22 22 2(1)()() xy yxxyvvv xyxy τ σσσσ ∂ ∂∂ +=−+− ∂∂ ∂∂ (v)
Also, from Eqs (1.6) and assuming that the body forces X and Y are zero
Suppose that the load in the steel bar is P st and that in the aluminium bar is P al . Then, from equilibrium
14
Solutions Manual
0 xzy xy τ σ ∂ ∂ += ∂∂ (vi) 0 yxy yx ∂∂στ += ∂∂ (vii) Differentiating Eq. (vi) with respect to x and Eq. (vii) with respect to y and adding gives 222 2 22 0 xxyyxy yxxyxy τστ σ ∂∂∂ ∂ +++= ∂∂∂∂∂∂ or 22 2 22 2 xyy x xyxy τσ σ ∂∂ ∂ =−+ ∂∂ ∂∂ Substituting in Eq. (v) 2 2 22 222 2 (1) ()() xy vyxxy vv xyxy σ σ σσσσ ∂ ∂ ∂∂ −++=−+− ∂∂∂∂ so that 22 2 2 22 222222 (1) xyyy xxvv xyxyxy σσ σ σ σσ ∂∂ ∂ ∂ ∂∂ −++=+−+ ∂∂∂∂∂∂ which simplifies to 22 22 2222 0 yy xx xyxy σσ σσ ∂∂ ∂∂ +++= ∂∂∂∂ or 22 22 ()0 xy xy σσ ∂∂ ++= ∂∂ S.1.9
stal PPP += (i) From Eq. (1.40) st al st al stst alal PP AEAE εε = =
Since the bars contract by the same amount
Due to the decrease in temperature in which no change in length is allowed the strain in the steel is αst T and that in the aluminium is αal T. Therefore due to the decrease in
The principal strains are given directly by Eqs (1.69) and (1.70). Thus
Solutions to Chapter 1 Problems 15
stal ststalal PP AEAE = (ii) Solving Eqs (i) and (ii) stst alal st ststalal ststalal al AEAE PPPP AEAEAEAE = = ++ from which the stresses are st al st al ststalal ststalal EE PP AEAEAEAE σσ = = ++ (iii) The areas of cross-section are 2 22 22 st al 75 (10075) 4417.9mm 3436.1mm 44 AAππ ×− == = = Substituting in Eq. (iii) we have 6 2 st 10200000 172.6N/mm(compression) (4417.92000003436.180000) σ × = = ×+× 6 2 al 1080000 69.1N/mm(compression) (4417.92000003436.180000) σ × = = ×+×
temperature 2 ststst 2000000.000012150360.0N/mm(tension) ET σα==××= 2 alalal 800000.00000515060.0N/mm(tension) ET σα==××= The final stresses in the steel and aluminium are then 2 st (total)360.0172.6187.4N/mm(tension) σ =−= 2 al (total)60.069.19.1N/mm(compression) σ =−=− S.1.10
22 I 11 (0.0020.002)(0.0020.002)(0.0020.002) 2 2 ε =−++−++++
The principal directions are given by Eq. (1.71),
S.1.11
The principal strains at the point P are determined using Eqs (1.69) and (1.70). Thus
Since P lies on the neutral axis of the beam the direct stress due to bending is zero. Therefore, at
and
Now subtracting
16 Solutions Manual i.e. I 0.00283 ε =+ Similarly II 0.00283 ε =−
i.e. 2(0.002)0.0020.002 tan2 1 0.0020.002 θ −+− = =− + Hence 245or135 θ =−°+° and 22.5or67.5 θ =−°+°
226 I 11 (22245)(222213)(21345)10 2 2 ε =−++−++−−× i.e. 6 I 94.010 ε =× Similarly 6 II 217.010 ε =−× The principal stresses follow from Eqs (1.67) and (1.68). Hence 6 I 2 31000 (94.00.2271.0)10 1(0.2) σ =−×× i.e. 2 I 1.29N/mm σ = Similarly 2 II 814N/mm σ =−
P, σ x =7 N/mm2
σ y = 0.
Eq. (1.12)
22 III 4 xxyσσστ −=+
from (1.11)
from which
The shear force at P is equal to Q so that the shear stress at P is given by
from which
Solutions to Chapter 2 Problems
S.2.1
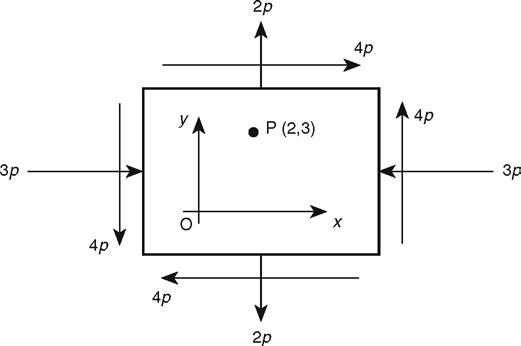
The stress system applied to the plate is shown in Fig. S.2.1. The origin, O, of the axes may be chosen at any point in the plate; let P be the point whose coordinates are (2, 3). Fig.
Solutions to Chapter 2 Problems 17 i.e. 1.298.147422xyτ +=+
τxy =
N/mm2
3.17
. 3 3.17 2150300 xy Q τ == ××
95100N95.1kN. Q ==
S.2.1
σ z = 0 323.5 x ppp v EEE ε =−−=− (i) 332.75 y ppp v EEE ε =−−=− (ii) Hence, from Eqs (1.27) 1 3.5 3.5 sothat () uppuxfyxEE ∂ =−=−+ ∂ (iii)
From Eqs (1.42) in which
Since the origin of the axes is fixed in space it follows that when x=y= 0,u=v= 0 Hence, from Eqs (vii) and (viii), B=D= 0. Further, the direction of Ox is fixed in space so that, wheny = 0, ∂v/∂x = 0. Therefore, from Eq. (viii), C = 0. Thus, from Eqs
and (vii), when x = 0.
and (viii) now become
18 Solutions Manual
f 1 (y)
2 2.75 2.75 sothat ()yEppyfx E υ υ ∂ ==−+ ∂ (iv)
which f 2 From the last of Eqs (1.52) and Eq. (1.28)
x)
a function of x 21()() 4 (fromEqs(iv)and(iii)) xy pufxfy Gxyxy υ γ ∂∂ ∂∂ ==+=+ ∂∂∂∂ Suppose 1 () fyA y ∂ = ∂ then 1 () fyAyB =+ (v) in which A and B are constants. Similarly, suppose 2 () fxC x ∂ = ∂ then 2 () fxCxD =+ (vi) in which C and D are constants. Substituting for f 1 (y) and f 2 (x) in Eqs (iii) and (iv) gives 3.5 p uxAyB E =−++ (vii) and 2.75EpyCxD υ =++ (viii)
where
is a function of y. Also
in
(
is
4 upA yG ∂ == ∂ Eqs (vii)
3.54pp uxy EG =−+ (ix)
(1.28)
The point P therefore moves at an angle α to the x axis given by S.2.2
An Airy stress function, φ , is defined by the equations (Eqs (2.8)):
and has a final form which is determined by the boundary conditions relating to a particular problem.
and the biharmonic equation (2.9) is satisfied. Further
The distribution of shear stress in a rectangular section beam is parabolic and is zero at the upper and lower surfaces. Hence, when y = ±d/2,
= 0. Thus, from Eq.
Solutions to Chapter 2 Problems 19 2.75 p Ey υ = (x) From Eq. (1.50), G
E/2(1 +ν) =E/2.5 and Eq.
becomes (3.510) p uy E =−+ (xi) At the
(2, 3) 23 (fromEq.(xi)) p u E = and 8.25 (fromEq.(x)) p E υ =
=
(ix)
point
222 22 xyxyxy yx φφφ σστ ∂∂∂ ===− ∂∂ ∂∂
Since 33 AyByxCyx φ =++ (i) 444 4422000 xyxy φφφ ∂∂∂ === ∂∂∂∂
2 2 66 xAyByx y φ σ ∂ ==+ ∂ (ii) 2 2 0 y x φ σ ∂ == ∂ (iii) 2 2 3 xyByC xy φ τ ∂ =−=−− ∂∂ (iv)
τ xy
2 4 3 C B d = (v)
(iv)
The resultant shear force at any section of the beam is –P. Therefore
from Eq. (iv)
Substituting for B from Eq. (v) gives
It now follows from Eqs (v) and (vi) that
At the free end of the beam where
for any value of y. Therefore, from Eq.
Then, from Eq. (ii)
Equation (ix) is the direct stress distribution at any section of the beam given by simple bending theory, i.e.
where M = P (l –x) and I = td3
The shear stress distribution given by Eq. (iv) is
20 Solutions
Manual
/2 /2 d d dxy tyP τ =− ∫
xy
/2 2 /2 (3)d d d ByCtyP −−=− ∫
3 2 82 BdCd tP +=
Substituting for τ
which gives
3 2 P C td = (vi)
3 2 P B td = (vii)
x
l
σ x 6A + 6Bl = 0 = 0
3 2 Pl A td = (viii)
33 1212 x PlP yxy tdtd σ =− or 3 12() x Plx y td σ = (ix)
=
the bending moment is zero and thus
(ii) whence
x My I σ =
/12. 2 3 63 2 xy PP tdytd τ =−
Engineering
5th Edition Megson Solutions Manual Full Download: http://testbanktip.com/download/aircraft-structures-for-engineering-students-5th-edition-megson-solutions-manual/ Download all pages and all chapters at: TestBankTip.com
Aircraft Structures for
Students















