Beginning and Intermediate Algebra with Applications and Visualization 3rd Edition Rockswold Solutio

BeginningandIntermediateAlgebrawithApplicationsandVisualization3rdEditionRockswoldSolutionsManual FullDownload:http://testbanktip.com/download/beginning-and-intermediate-algebra-with-applications-and-visualization-3rd-edition-rockswold-solutions-manual/

Chapter 2: Linear Equations and Inequalities

Section 2.1 Introduction to Equations

17. 505505055; xxxx +=⇒+−=−⇒+=−⇒=− To check your answer, substitute 5 for x in the original equation, 550.−+= This statement is true. Thus, the solution 5 x =− is correct.

18. 373373044; xxxx +=⇒+−=−⇒+=⇒= To check your answer, substitute 4 for x in the original equation, 437. += This statement is true. Thus, the solution 4 x = is correct.

19. 1231212312099 aaaa −=−⇒−+=−+⇒+=⇒=

20. 191119191119088 aaaa −=−⇒−+=−+⇒+=⇒=

21. 9898881701717 yyyyy =−⇒+=−+⇒=+⇒=⇒=

22. () 9723972323231200120 yyyy =−+⇒+=+−+⇒=+⇒=

23. 131333171717 0 525222101010 zzzzz =−⇒+=−+⇒=+⇒=⇒=

24. 31331355 0 42442444 zzzz ⎛⎞⎛⎞ +=−⇒+−+=−+−⇒+=−⇒=− ⎜⎟⎜⎟

25. 0.84.30.80.84.30.805.15.1 tttt −=⇒−+=+⇒+=⇒= 26. 1.230.021.231.230.021.2301.211.21 yyyy −=−⇒−+=−+⇒+=⇒=

Copyright ©2013 Pearson Education, Inc

Downloadallpagesandallchaptersat:TestBankTip.com

Chapter 2 Linear Equations and Inequalities

27. 414414033 xxxx +=⇒−+=−⇒+=−⇒=−

28. 162161621601818 xxxx +=−⇒−+=−−⇒+=−⇒=−

29. 1111222 110 3333333 yyyyy =+⇒−=−+⇒=+⇒=⇒=

30. 77111111 22220 22222 yyyyy =−+⇒+=−++⇒=+⇒=⇒=

37. 515 5153 55 x xx =⇒=⇒=

38. 28 284 22 x xx −=⇒=⇒=−

39. 70 700 77 x xx −=⇒=⇒=

40. 250 2500 2525 x xx =⇒=⇒=

41. 355 35577 55 a aaa −=−⇒=⇒=⇒=

42. 324 32488 44 a aaa −=−⇒=⇒=⇒=

43. 183 18366 33 a aaa −=⇒=⇒−=⇒=−

44. 7010 701077 1010 a aaa −=⇒=⇒−=⇒=−

45. 132132 3 221221 xxx =⇒⋅=⋅⇒=

46. 3543545 4834836 xxx =⇒⋅=⋅⇒=

47. 12515255 25222544 zzzz =⇒⋅=⋅⇒=⇒=

48. 313881 66 484118 zzzz ⎛⎞⎛⎞ −=−⇒−⋅−=−⋅−⇒=⇒=

Copyright ©2013 Pearson Education, Inc

55. (a) See Figure 55.

(b) Let R represent total rainfall and let x represent the number of hours past noon. Start with 3 inches of rain and then add 1 , 2 or 0.5, inches per hour after noon, 30.5, or equivalently 0.53.xRRx +==+

(c) At 3 pm, 3. x = Substituting x with 3 in the formula, 0.5334.5 inches. RR=⋅+⇒= This answer agrees with the table from part (a).

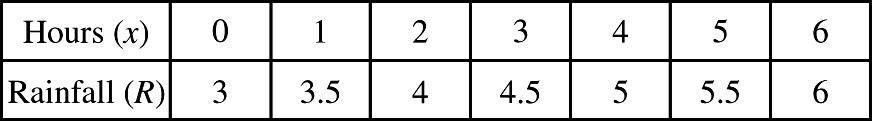
(d) At 2:15 pm, 2.25. x = Substituting x with 2.25 in the formula, 0.52.2534.125 inches. RR =⋅+⇒=

56. (a) See Figure 56.

(b) Let T represent the temperature and let x represent the number of hours past midnight. Since the temperature increases 10ºF per hour, 10.Tx =

(c) At 5 am, 5. x = Substituting x with 5 in the formula, 10550ºF.TT=⋅⇒= This agrees with the table from part (a).

(d) At 2:45 am, 2.75. x = Substituting x with 2.75 in the formula, 102.7527.5ºF.TT=⋅⇒=

57. (a) Let L be the length of the football fields and x be the number of fields. Because each field x contains 300 feet, 300.Lx =

(b) Substitute L with 870. Then 870300. x =

(c) 870300870

8703002.9 300300300 x xxx =⇒=⇒=⇒=

58. (a) Let A represent the number of acres and let S represent the number of square feet. Because each acre contains 43,560 square feet, let 43,560. SA =

(b) Substitute S with 871,200. Then, 871,20043,560. A =

Figure 55

Figure 56

Chapter 2 Linear Equations and Inequalities

59. The formula for this scenario is 0.3 Tx = where x is in days and T is in millions. To find the number of days needed for Twitter to add 15 million new accounts, replace the variable T in the formula with 15. 150.3 0.3150.350 0.30.3 x Txxx =⇒=⇒=⇒= At this rate it takes 50 days to add 15 million accounts.

60. The formula for this scenario is 14,000 Wx = where x is in days and W is the number of visitors. To find the number of days for the Web site to add 98,000 new visitors, replace the variable W in the formula with 98,000.

98,00014,000 14,00098,00014,0007 14,00014,000 x Wxxx =⇒=⇒=⇒= At this rate it takes 7 days to add 98,000 new visitors.

61. a) The latitude of Winnipeg to the nearest degree is 50 N

b) The sun at the equator will be approximately 325 5.7 57 ≈ times as intense as they are in Winnipeg on March 21st.

62. a) The latitude of Columbus, Ohio to the nearest degree is 40 N

b) The sun in Limon, Costa Rica will be approximately 275 1.4 199 ≈ times as intense as they are in Columbus on June 21st

63. Let x represent the cost of the car to obtain the equation 0.071750. x = Then the solution is

64. Let S represent the employee’s current salary to obtain the equation 1.0658,300. S = Then the

Section 2.2 Linear Equations

Copyright ©2013 Pearson Education, Inc

10. 214 is a linear equation. x −+= 21421444230. xxx −+=⇒−+−=−⇒−−=

2 and 3. ab=−=−

11. 11 0 is a linear equation. and 0. 22 xab ===

12. 33 0 is a linear equation. and 0. 44 xab −==−=

13. 2 4611 x −= is not a linear equation because it cannot be written in the form 0. axb+= It has a non-zero term containing 2 x

14. 2 24 xx −+= is not a linear equation because it cannot be written in the form 0. axb+= It has a non-zero term containing 2 . x

15. 6 42 x −= is not a linear equation because it cannot be written in the form 0. axb+= It has the variable x in the denominator.

16. 210 x −= is not a linear equation because it cannot be written in the form 0. axb+= It has a nonzero term containing . x

17. 1.10.91.8 is a linear equation.

1.10.91.81.10.91.81.81.81.10.90.1.1 and 0.9. x xxxab += +=⇒+−=−⇒−===−

18. 5.73.46.8 is a linear equation.

5.73.46.85.73.46.86.86.85.73.40.5.7 and 3.4.

xxxab

19. () 230 x −= is a linear equation. Use the distributive property to obtain 260. 2 and 6. xab −===−

20. () 1 40 2 x += is a linear equation. Use the distributive property to obtain 11 20. and 2. 22 xab +===

21. 321 x += is not a linear equation because it cannot be written in the form 0. axb+= It has a nonzero term containing . x

22. 3 34xx = is not a linear equation because it cannot be written in the form 0. axb+= It has a nonzero term containing 3 . x

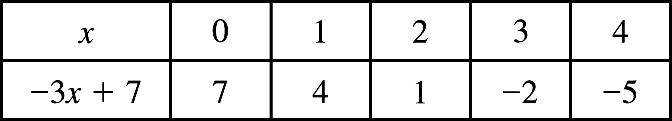
23. For 1, substitute 1 for and solve:134. xx =−−−−=−

For 0, substitute 0 for and solve:033. xx =−=−

For 1, substitute 1 for and solve:132. xx =−=−

For 2, substitute 2 for and solve:231. xx =−=−

For 3, substitute 3 for and solve:330. xx =−=

Copyright ©2013 Pearson Education, Inc

Chapter 2 Linear Equations and Inequalities

See Figure 23. From the table, we see that the equation 31 is true when 2. xx −=−= Therefore, the solution to the equation 31 is 2. xx−=−=

24.

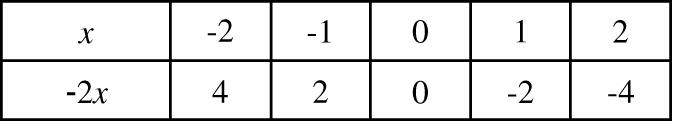
() For 2, substitute 2 for and solve:224. xx =−−−−=

() For 1, substitute 1 for and solve:212. xx =−−−−=

() For 0, substitute 0 for and solve:200. xx =−=

() For 1, substitute 1 for and solve:212. xx =−=−

25.

()

For 2, substitute 2 for and solve:2274. xx =−+=−

See Figure 24. From the table, we see that the equation 20 is true when 0. xx −== Therefore, the solution to the equation 20 is 0. xx −==

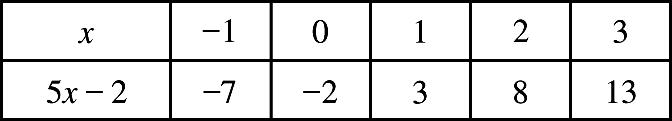
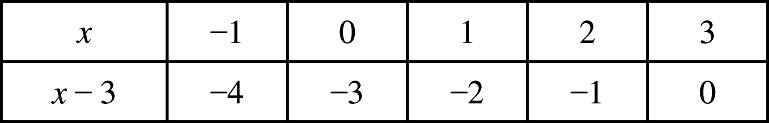
() For 0, substitute 0 for and solve:307077. xx =−+=+=

() For 1, substitute 1 for and solve:317374. xx =−+=−+=

For 2, substitute 2 for and solve:327671. xx =−+=−+=

()

() For 3, substitute 3 for and solve:337972. xx =−+=−+=−

() For 4, substitute 4 for and solve:3471275. xx =−+=−+=−

See Figure 25. From the table, we see that the equation 371 is true when 2. xx −+== Therefore, the solution to the equation 371 is 2. xx −+==

26. () For 1, substitute 1 for and solve:512527. xx =−−−−=−−=−

() For 0, substitute 0 for and solve:502022. xx =−=−=−

() For 1, substitute 1 for and solve:512523. xx =−=−=

() For 2, substitute 2 for and solve:5221028. xx =−=−=

() For 3, substitute 3 for and solve:53215213. xx =−=−=

See Figure 26. From the table, we see that the equation 523 is true when 1. xx −== Therefore, the solution to the equation 523 is 1. xx−==

27.

() For 2, substitute 2 for and solve:422448. xx =−−−−=+=

For 1, substitute 1 for and solve:421426. xx =−−−−=+=

()

() For 0, substitute 0 for and solve:420404. xx =−=−=

Copyright ©2013 Pearson Education, Inc

Figure 23

Figure 24

Figure 25

Figure 26

28.

() For 1, substitute 1 for and solve:421422. xx =−=−=

() For 2, substitute 2 for and solve:422440. xx =−=−=

See Figure 27. From the table, we see that the equation 426 is true when 1. xx −==− Therefore, the solution to the equation 426 is 1. xx −==−

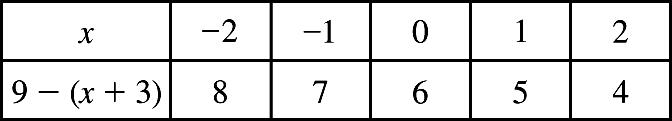
Figure 27

Figure 28

() For 2, substitute 2 for and solve:923918. xx =−−−−+=−=

For 1, substitute 1 for and solve:913927. xx =−−−−+=−=

()

() For 0, substitute 0 for and solve:903936. xx =−+=−=

For 1, substitute 1 for and solve: 913945. xx =−+=−=

()

() For 2, substitute 2 for and solve: 923954. xx =−+=−=

See Figure 28. From the table, we see that the equation () 934 is true when 2. xx −+==

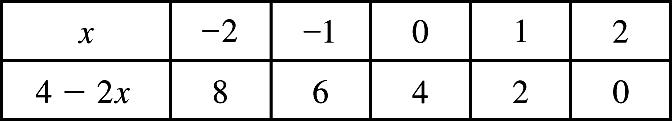
Therefore, the solution to the equation () 934 is 2. xx −+==

29. 1133 113 111111 x xx =⇒=⇒=

30. 515 5153 55 x xx −=⇒=⇒=−

31. 185181851823 xxx −=⇒−+=+⇒=

32. 33 853855533311 33 x xxxxx =+⇒−=−+⇒=⇒=⇒=⇒=

33. 1111 1131113114221428 2222 x xxxx −=⇒−+=+⇒=⇒⋅=⋅⇒=

34. 1111 393393644624 4444 x xxxx +=⇒+−=−⇒=⇒⋅=⋅⇒=

35. 11511 65565555115 555 x xxxx −=+⇒−−=+−⇒−=⇒=⇒=−

36. 357 31743147443575 77 x xxxx =−−⇒+=−−+⇒=−⇒=⇒=−

37. 32532252373727 zzzzzzzzzzz +=−⇒+−=−−⇒=−⇒−=−−⇒=−⇒ 277 222 z z =⇒=−

38. 5535553552555242 zzzzzzzzzzz −=−⇒−+=−+⇒=+⇒−=−+⇒−=⇒ 421 442 z z =⇒=−

Copyright ©2013 Pearson Education, Inc

Chapter 2 Linear Equations and Inequalities

39. 12633126633612391239 yyyyyyyyyy −=−⇒−+=+−⇒=−⇒+=−+⇒ 1339 13393 1313 y yy =⇒=⇒=

40. 1322231322222313203 yyyyyy −+=−⇒−+−=−−⇒−=−⇒ 1020 133203310202 1010 y yyyyyy −+=−+⇒−=⇒=⇒=−

41. () 499 4154454445449 444 x xxxxx −=⇒−=⇒−+=+⇒=⇒=⇒=

42. () 415 2271414141414114415 44 x xxxx −+=⇒−−=⇒−−+=+⇒−=⇒=⇒ 15 4 x =−

43. () 131513153535 xxxxxxxxxx −+=−⇒−−=−⇒−=−⇒−+=−+⇒ 255 25 222 x xx −=⇒=⇒=−

44. ()() 62710336214103928193 xxxxxx +−=−−⇒+−=−+⇒−=−⇒

23819335819588198527 xxxxxxx +−=−+⇒−=⇒−+=+⇒=⇒ 52727 555 x x =⇒=

45. ()() 5621256222562452662264 tttttttttt −=++⇒−=++⇒−=+⇒−−+=−++⇒ 31010 310 333 t tt

46. ()()

27552145539539959 tttttt −−−+=⇒−+−−=⇒−+=⇒−+−=−⇒ 344 34 333 t tt −=−⇒=⇒=

47.

3412221123242210722 zzzzzzzz −−+=+⇒−−−=+⇒−=+⇒

10772271029 zzzz −+=++⇒=+⇒ 899 10222989 888 z zzzzzz −=−+⇒=⇒=⇒=

48. ()()()

43121431222322 zzzzzzzz −+++=−+⇒−−++=−−⇒−=−−⇒ 2332232212222141 zzzzzzzzz −+=−−+⇒=−+⇒+=−++⇒=⇒ 411 444 z z =⇒= 49.

7.37.3

7.31.75.67.31.71.75.61.77.37.31

7.37.3 x xxxx −=⇒−+=+⇒=⇒=⇒=

5.53518.5516 8.58.5 x xxxx +=⇒=⇒=⇒=

51. 9.50.0510.51.059.510.50.0510.510.51.05 xxxxxx −−=+⇒−−−=−+⇒

200.051.05200.050.051.050.05201.1 2020 x xxx −−=⇒−−+=+⇒−=⇒=⇒ 0.055 x =−

Copyright ©2013 Pearson Education, Inc

201.1

52. 0.040.030.020.10.040.020.030.020.020.1 xxxxxx +=− 0.020.030.1 x +=−⇒

0.020.030.030.10.030.020.13 xx +−=−−⇒=−⇒ 0.020.13 6.5 0.020.02 x x =⇒=−

53. 1351335311 42428 2222222222 xxxxx −=⇒−+=+⇒=⇒⋅=⋅⇒=

54. () () 153155351212 442 444444444444 xxxxx ⎛⎞ −+=⇒−+−=−⇒−=−⇒−⋅−=−⋅−⇒= ⎜⎟

55. 31131131 84884888 xxx −+=⇒−=−+⇒−=−⇒ 83181 38833 xx

−−=−−⇒= ⎜⎟⎜⎟⎜⎟⎜⎟

⎝⎠⎝⎠⎝⎠⎝⎠

56. 11111141141111 34634634634464 xxxxxxxx +=−⇒++=−+⇒+=⇒+−=−⇒ 41341331 312431244816 xxxx =−⇒⋅=−⋅⇒=−⇒=−

57. () 421042202202220222 yyyyyyy −+=⇒−−=⇒−=⇒−+=+⇒=⇒ 22 1 22 y y =⇒=

58. () 15205510102051010102051010 yyyyyyyyy +−=−⇒+=−⇒++=−+⇒

202052020205202015

20204 y yyyy +=⇒+−=−⇒=−⇒=⇒=−

20153

59. 00 axbaxbbb +=⇒+−=− axb⇒=− axb aa ⇒= b x a ⇒=−

60. 11 00 xbxbbb aa −=⇒−+=+ 1 xb a ⇒= 1 axab a ⇒⋅=⋅ xab⇒=

61. 5515555101 xxxxxx =+⇒−=−+⇒=

Because the equation 01 = is always false, there are no solutions.

62. () 2326262622622666 xxxxxxxx −=−⇒−=−⇒−−=−−⇒−=−

Since the equation 66−=− is always true, there are infinitely many solutions.

63. 80 800 88 x xx =⇒=⇒= Thus, there is one solution.

64. 811 919181 888 x xxxxxxxx =+⇒−=−+⇒=⇒=⇒= Thus, there is one solution.

65. () 45345154415444415015 xxxxxxxxxxxx =+−⇒=+−⇒=+⇒−=−+⇒=

Because the equation 015 = is always false, there are no solutions.

66. () 515275152145215221471 xxxxxxxxx =−+⇒=−−⇒+=−+−⇒=⇒

711 777 x x =⇒= Thus, there is one solution.

Copyright ©2013 Pearson Education, Inc

Chapter 2 Linear Equations and Inequalities

67. ()() 527105301035105303030 xxxx +−+=⇒+−−=⇒=

Since the equation 3030 = is always true, there are infinitely many solutions.

68. ()() 42223104846108610210 xxxx +−+=⇒+−−=⇒−=⇒=

Because the equation 210 = is always false, there are no solutions.

69. ()321523215222152 xxxxxxxx −+=−⇒−−=−⇒−−=−⇒ 2221522215 xxxx −+−=−+⇒−=

Because the equation 215−= is always false, there are no solutions.

70. () 2552555555 xxxxxxxxxxxx −+=−⇒−−=−⇒−=−⇒−−=−−⇒ 55−=− Since the equation 55−=− is always true, there are infinitely many solutions.

71. (a) See Figure 71.

(b) Let D represent the distance from home and x represent the number of hours. Then 48. Dx =+

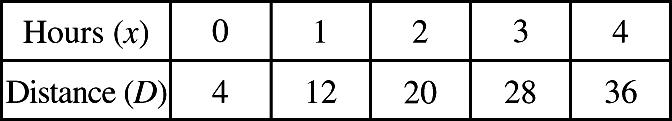
(c) Substitute 3 for x. Then, () 48328 D =+= miles. This agrees with the value found in the table.

(d) Using the formula 48, Dx =+ substitute 22 for D. Then, 2248. x =+ Then, solving for x:

1889

xxxx −=−+⇒=⇒=⇒=⇒= Thus, the bicyclist is 22 miles from home after 2 hours and 15 minutes.

Figure 71

884 x

2244481882.25 hours.

72. (a) Let D represent distance from home and x represent hours. Note that each hour spent running decreases the distance from home by 6 miles. Thus, set the formula to 166. Dx =−

(b) Using the formula, substitute 1.5 for x and solve for D () 1661.51697 miles. DDD =−⇒=−⇒=

(c) Substitute 5.5 for D and solve for x. 5.51665.5161616610.56 xxx =−⇒−=−−⇒−=−⇒

10.56 1.751.75 66 x xx =⇒=⇒= Thus, the athlete is 5.5 miles from home after 1 hour and 45 minutes of running.

73. Using the formula, substitute 1730 for I and solve for x. 1730241482,440 x =−⇒

1730482,440241482,440482,440 x +=−+

484,1702412009 241241 x xx ⇒=⇒=⇒≈

74. Using the formula, substitute 970 for N and solve for x. 9704283,19797083,1974283,19783,197 xx =−⇒+⇒−+

84,16742 84,167422004 4242 x xx ⇒=⇒=⇒≈

Copyright ©2013 Pearson Education, Inc

75. Using the formula, substitute 1.5 for N and solve for x

1.50.0358.621.558.620.0358.6258.62 xx =−⇒+=−+

60.120.03

0.030.03 x xx ⇒=⇒=⇒=

60.120.032004

76. Using the formula, substitute 6.6 for C and solve for x. 6.60.35684 x =−⇒

690.60.35

6.66840.35684684690.60.351973.143 0.350.35 x xxx +=−+⇒=⇒=⇒≈⇒

1973.143 x ≈ Thus, the cost reached $6.6 billion sometime in the year 1973.

77. Using the formula, substitute 2841 for H and solve for x. 28413369,105 x =−+⇒

6626433

284169,1053369,10569,10566264332008 3333 x xxx −=−−+⇒−=−⇒=⇒=

Thus, the number of hospitals reached 2841 in 2008.

78. Using the formula, substitute 2504 for F and solve for x. 25043465,734 x =−⇒

68,23834

250465,7343465,73465,73468,238342007 3434 x xxx +=−+⇒=⇒=⇒=

Thus, the average home size reached 2504 square feet in 2007.

Checking Basic Concepts Sections 2.1 and 2.2

1. (a) 3 420 x −= is not linear because it cannot be written in the form 0. axb+= It has a non-zero term containing 3 x

(b) () 214 x += is a linear equation. Use the distributive property to obtain 224, x += then 22444 x +−=− 220. x ⇒−= 2 a = and 2. b =−

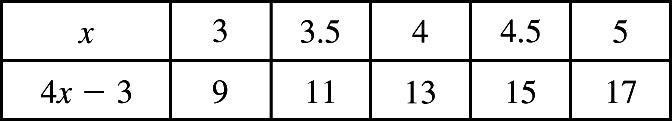
2. For 3, x = substitute 3 for x and solve: () 4331239 −=−=

For 3.5, x = substitute 3.5 for x and solve: () 43.5314311 −=−=

For 4, x = substitute 4 for x and solve: () 44316313 −=−=

For 4.5, x = substitute 4.5 for x and solve: () 44.5318315 −=−=

For 5, x = substitute 5 for x and solve: () 45320317 −=−=

See Figure 2. To solve 4313, x −= the table tells us that when 4, 4313.xx=−= Figure 2

Copyright ©2013 Pearson Education, Inc

Chapter 2 Linear Equations and Inequalities

3. (a) 126121261218 xxx −=⇒−+=+⇒= To check the answer, substitute 18 for x in the original equation 126. x −= 1812666. −=⇒= Since this is true 18 x = is correct.

(b) 31431441 483483246 zzzz =⇒⋅=⋅⇒=⇒=

t tttt +=⇒+−=−⇒=⇒=⇒=

(d) ()() 5223452412392123 xxxxxx −−=−⇒−+=−⇒−=−⇒ 9231233912991293 xxxxxxx −+=−+⇒+=⇒−+=−⇒=

4. (a) 55 5656551. 55 x xxxxxxxx −=⇒−−=−⇒−=⇒=⇒−= Thus, the equation has one solution.

(b) () 25102210102221010221010 xxxxxxxx −−=−⇒−+=−⇒−++=−+⇒=

Since 1010 = is always true, the equation has infinitely many solutions.

(c) ()11111111 xxxxxxxx −−=−−⇒−+=−−⇒−++=−+−⇒=−

Since this is never true, the equation has no solutions.

5. (a) Let D represent distance from home and x represent hours driven. Note that the driver is initially 300 miles from home and that each hour driven the driver gets closer to home by 75 miles. Thus, the formula is 30075. Dx =−

(b) Since the distance from home, when the driver is home, is 0, use the formula and set D equal to 0. Thus, 030075. x =−

(c) 75 300 0300750753007575753004 hours. 7575 x xxxxxx =−⇒+=−+⇒=⇒=⇒=

Section 2.3 Introduction to Problem Solving

1. Check your solution.

Copyright ©2013 Pearson Education, Inc

10. increase; decrease

11.rt

12. distance; time

13. Let t represent the number. 2122212210 ttt +=⇒−+=−⇒=

14. 22 27927797221 22 x xxxx +=⇒+−=−⇒=⇒=⇒=

15. () 24552451205551204120 55 xx xxxxxxxxx =−⇒⋅=−⇒=−⇒−=−−⇒−=−⇒

4120 30 44 x x =⇒=

16. 25125 251255 2525 x xx =⇒=⇒=

17. 55 7272514551459 22 xx xxx ++ =⇒⋅=⋅⇒+=⇒+−=−⇒=

18. ()() 85885831133 xxxxx −=⇒−−=−⇒−=−⇒−−=−−⇒=

19. 17217234 22 xx x =⇒⋅=⋅⇒=

20. 595 59519 55 x xx =⇒=⇒=

21. Let the smallest natural number be represented by x ()()12963396xxxx ++++=⇒+=⇒

393 33396339331 33 x xxx +−=−⇒=⇒=⇒= Thus, the numbers are 31, 32 and 33.

22. Let x represent the smallest integer, ()()1212333123xxxx ++++=−⇒+=−⇒

3126

3331233312642 33 x xxx +−=−−⇒=−⇒=⇒=−

Thus, the numbers are 42, 41 and 40.

23. 3102 310234 33 x xx =⇒=⇒=

24. 1821821818 xxxxxxxx +=⇒−+=−⇒=⇒=

25. 324 52245222243248 33 x xxxxxxxx =+⇒−=−+⇒=⇒=⇒=

26. 218 3183182189 22 x xxxxxxxx =−⇒−=−−⇒=−⇒=⇒=−

27. 666126 187187612621 7766 xxx xx =⇒⋅=⋅⇒=⇒=⇒=

28. 2222 45452220222202222 55 xx xxx =⇒⋅=⋅⇒−=⇒−+=+⇒=⇒

222 11 22 x x =⇒=

Copyright ©2013 Pearson Education, Inc

29. Let x represent the child’s current age.

10231023103 xxxxxxx +=+⇒−+=−+⇒=+ 1033377 xxx ⇒−=+−⇒=⇒=

30. Let x be the age of the daughter then 215 x + is the age of the mother.

The equation is 49215. x =+

342

4921549152151534217and21549 22 xxxxxx =+⇒−=+−⇒=⇒=⇒=+=

31.Let x be the previous weight of the individual.

() 11 301103303110 33 xxxx −=+⇒−=+⎛⎞ ⎜⎟ ⎝⎠

2903302909033090 xx −=⇒−+=+

390330390330 xxxxxx ⇒−=+⇒−−=−+⇒

2420 2420210lb 22 x xx ⇒=⇒=⇒=

32. Let x be the person’s weight before gaining 25 pounds. The equation is 252115xx+=−

2521152511525115115115140 xxxxxxx −+=−−⇒=−⇒+=−+⇒= pounds

33. Let x be the number of waste sites in Washington.

2422242222262 xxx =−⇒+=−+⇒= 262 1313 22 x xx ⇒=⇒=⇒=

34. Let x be the number of unhealthy air quality days in San Diego.

993

1003110013119933333 33 x xxxxx =+⇒−=+−⇒=⇒=⇒=⇒=

35. Let x be the number of reptiles on the endangered species list.

36.

922129212212128024040 22 x xxxxx =+⇒−=−+⇒=⇒=⇒=⇒=

802

844 8438442121and363 44 x xxxxxx =+⇒=⇒=⇒=⇒==

There are 63,000 millionaires in Kentucky and 21,000 millionaires in Rhode Island.

37. 15622501562156225015621812 xxx −=⇒−+=+⇒= million

38.

5002 311218931118921891895002250 22 x xxxx =−⇒+=−+⇒=⇒=⇒=⇒

250 x = billion kilowatt-hours

39. 274 247024470427437 22 x xxxx −=⇒−+=+⇒=⇒=⇒=

There were 37,000 cosmetic surgeries performed on persons under age 18.

40. 368132236822132222 xx =−⇒+=−+

39013

390133030 1313 x xxx ⇒=⇒=⇒=⇒= thousand

41. 1062(5)210621021064101061041010

964 9642424 44

xxxxxx x xxx

=++⇒=++⇒=+⇒−=+−⇒ =⇒=⇒=⇒=

Copyright ©2013 Pearson Education, Inc

=++++⇒=+⇒−=+−⇒=⇒

xxxxxx x xx =⇒=⇒=

43. 6222(7)62221462414621441414

484 4841212and719 44

xxxxxx x xxxx

=++⇒=++⇒=+⇒−=+−⇒ =⇒=⇒=⇒=+=

The length is 19 inches and the width is 12 inches.

44. 102(15)(6)1023211022132121 1233

=+−+−⇒=−⇒+=−+⇒ =⇒=⇒=⇒=−=−=

xxxxx x xxxxx

12334141,635,and1526 33

The measures are 26, 35, and 41 feet.

45. 170(210)1703101701031010 1803

18036060and210110 33

=+−⇒=−⇒+=−+⇒ =⇒=⇒=⇒=−=

xxxx x xxxx

Therefore, Facebook had 60 million users and MySpace had 110 million users.

46. 225400225225400225175 xxx +=⇒+−=−⇒=

There were 175 million Facebook users in February 2009.

47. 248,000(52,000)248,000252,000248,00052,0002

300,0002

=⇒=⇒=⇒=−=

52,00098,000 22 xxxx x xxxx =+−⇒=−⇒+=⇒

49. 37 37% 100 = 37%370.010.37 =×= 50. 5213413 52% 10025425 === ⋅ 52%520.010.52 =×= 51. 14837437 148% 10025425 === 148%1480.011.48 =×= 52. 25263463 252% 10025425 === 252%2520.012.52 =×= 53. 6.96.91069 6.9% 100100101000 ==⋅= 6.9%6.90.010.069 =×= 54. 8.18.11081 8.1% 100100101000 ==⋅= 8.1%8.10.010.081 =×= 55. 0.050.051005151 0.05% 10010010010,000200052000 ==⋅=== 0.05%0.050.010.0005 =×=

Copyright ©2013 Pearson Education, Inc

56. 0.120.1210012343 0.12% 10010010010,000250042500 ==⋅===

0.12%0.120.010.0012 =×=

57. 0.450.45100%45% =×=

58. 0.080.08100%8% =×=

59. 1.81.8100%180% =×=

60. 2.972.97100%297% =×=

61. 0.0060.006100%0.6% =×=

62. 0.00010.0001100%0.01% =×=

63. 2 0.40.4100%40% 5 ==×=

64. 1 0.3330.333100%33.3% 3 ==×=

65. 3 0.750.75100%75% 4 ==×=

66. 7 0.350.35100%35% 20 ==×=

67. 5 0.8330.833100%83.3% 6 ==×=

68. 53 1.061.06100%106% 50 ==×=

69. Let B represent voters in 2008 and let A represent voters in 1980. Then, the percent change in the number of voters is 132.686.546.1 0.533 or about 53.3%.

86.586.5 BA A ==≈

70. Let B represent the number of master’s degrees received in 2010 and let A represent the number of master’s degrees received in 2005. Then, the percent change in the number of master’s degrees received is 659,000594,00065,000 0.109or about 10.9%.

594,000594,000 BA A ==≈

71. Calculate the value of 4% of 950, then add the value to 950. Thus, () 4% of 950.0495038. == Then, 95038$988 per credit. +=

72. Calculate the value of 8% of 125, then add the value to 125. Thus, () 8% of 125.0812510. == Then, 12510$135 per credit. +=

73. To calculate the total area of Wisconsin, let x represent the unknown number and note that 13.6 is 38% of the unknown number. Then, 0.3813.6 0.3813.635.8. 0.380.38 x xx =⇒=⇒≈ Thus, the total area of Wisconsin is about 35.8 million acres.

Copyright ©2013 Pearson Education, Inc

74. Let x be the number of female military personnel in 2008.

75. The percent change from $1.20 to $1.50 is, 1.501.20 10025 1.20 ⋅= Thus, the percent change from $1.20 to $1.50 is 25%. The percent change from $1.50 to $1.20 is 1.201.50 10020 1.50 ⋅=−

Thus, the percent change from $1.50 to $1.20 is 20%.

76. Given the percent change is 30% when the amount increases from 1A to 2 , A then 21

⎛⎞ ⇒=⇒−=⇒−+=+ ⎜⎟

0.30.30.3 AA AAAAAAAAAA

() 2112121 0.30.311.3. AAAAAAA ⇒=+⇒=+⇒=

Then, if the amount decreases from 2A back to 1 , A () 1 12111 2111

11.3 1.3 0.3 0.231. 1.31.31.3 A AAAAA AAAA ===⋅≈−

So the percent charge is not 30%, it is about 23.1%. 77. 428 miles drtdd =⇒=⋅⇒=

drtttt =⇒=⇒=⇒=⇒= 83. Given that the distance traveled (d) is 255 and that the time spent traveling (t) is 4.25 hours, calculate the speed of the car (r).

Then,

r drtrrr =⇒=⋅⇒=⇒=⇒=

Given the distance and the time, calculate the speed. Then,

mileshour.

Copyright ©2013 Pearson Education, Inc

85. Let the slower runner be standing still. Then, the faster runner will be traveling at 02 mph. + Then, this problem is equivalent to solving how long it takes the faster runner to travel 3 4 of a mile. Using the drt = formula: 3312133 2. 4421288 tttt =⇒⋅=⋅⇒=⇒= So, in 3 8 hour the faster runner will be 3 4 mile ahead of the slower runner.

86. Since the athlete runs 1 3 of an hour at 6 mph, the athlete runs 1 62 3 ⋅= miles in the first 1 3 of an hour. Since the athlete runs a total of 8 miles, the athlete runs 826 −= miles in the remaining 12 1 33 −= of an hour. Using the formula: drt = 2323 6699. 3232 rrrr =⋅⇒⋅=⋅⋅⇒=⇒= Therefore, the second speed is 9 miles/hour.

87. Let t represent the amount of time spent running 5 mph. Since the total time spent running was 1.1 hours, then 1.1 t represents the amount of time running at 8 mph. Using the drt = formula, the distance run will equal the sum of () 5 and 81.1.tt

Thus, () 7581.1758.8878.83 ttttt =+−⇒=+−⇒=−⇒ 1.8 378.833378.83778.8731.80.6. 3 ttttttt +=−+⇒+=⇒+−=−⇒=⇒==

Therefore, the athlete ran at 5 mph for 0.6 hour and ran at 8 mph for () 1.10.60.5 hour. −=

88. The distance the bus travels is 160295455 += miles. The bus travels at 70 mph. Then, 45570 455706.56.5. 7070 t ttt =⇒=⇒=⇒= Therefore, it will take the bus 6.5 hours to be 295 miles west of the border.

89. Since the plane is already 300 miles west of Chicago, it will have to fly 21753001875 −= miles to be 2175 miles west of Chicago. The plane is traveling at 500 mph. Then, 1875500

18755003.753.75. 500500 t ttt =⇒=⇒=⇒= Therefore, it will take the plane 3.75 hours to be 2175 miles west of Chicago.

90. Let x represent the speed of the slower car and 6 x + represent the speed of the faster car. Then, the distance of 171 miles will equal the speed of the slower car multiplied by the time added to the speed of the faster car multiplied by the time.

Therefore, ()()() 1711.561.51711.51.59 xxxx =++⇒=++⇒

1623

17139171939916235454. 33 x xxxxx =+⇒−=+−⇒=⇒=⇒=⇒=

Thus, 54 mph is the speed of the slower car and 54660 += mph is the speed of the faster car.

Copyright ©2013 Pearson Education, Inc

91. Let x represent the amount of water that should be added. Note that there is no salt in pure water and that we will add the amount of pure water to the 3% salt solution to obtain a 1.2% solution. Therefore, set up the equation so that the amount of salt on both sides of the equation is equal. Thus, ()()()()

Therefore, 30 ounces of water should be added.

92. Let x represent the amount of water that should be added. Note that there is no hydrochloric acid in pure water and that we will add the amount of pure water to the 15% solution to obtain a 2% solution. Therefore, set up the equation so that the amount of hydrochloric acid on both sides of the equation is equal. Thus, ()()()() 0.00500.15500.020.007.50.021 xxxx+=+⇒+=+⇒

x

Therefore, 325 ml of water should be added.

93. Let x represent the amount of the loan at 6% and let 1000 x + represent the amount of the loan at 5%. The total interest for one year is $215 and this is the sum of the interest paid on the two loans. Therefore: () 0.060.0510002150.060.0550215 xxxx ++=⇒++=⇒

Therefore, the amount of the loan at 6% interest is $1500 and the amount of the loan at 5% interest is 15001000$2500. +=

94. Let x represent the interest rate for the $3000 loan and let 0.03 x represent the interest rate for the $5000 loan. The total interest cost for the year will equal the sum of the interest for each loan. Therefore: () 300050000.0355030005000150550 xxxx +−=⇒+−=⇒

Therefore, the interest rate for the $3000 loan is 8.75% and the interest rate for the for the $5000 loan is 0.08750.030.0575 or 5.75%. −=

95. Let x represent the amount of 70% antifreeze. Then, the 45% antifreeze mixture is the sum of the 70% mixture and the 30% mixture. Therefore, ()()() 0.7100.3100.45 xx +=+⇒

0.730.454.5 xx+=+⇒ 0.7330.454.53 xx +−=+−⇒

0.70.451.50.70.450.450.451.5 xxxxxx =+⇒−=−+⇒

0.251.56. 0.250.25 x xx =⇒=⇒=

Therefore, 6 gallons of 70% antifreeze should be mixed with 10 gallons of 30% antifreeze to obtain the 45% mixture.

Copyright ©2013 Pearson Education, Inc

96. Since there is a total of 50 gallons of mixture, let x represent the gallons of 65% antifreeze. Then 50 x represent the 20% antifreeze. Then: ()() 0.650.2050500.56

Therefore, 40 gallons of 65% antifreeze should be mixed with 10 gallons of 20% antifreeze.

97. Let x represent the amount of 2.5% cream. Then, the 1% cream is the sum of the 2.5% cream and the base cream ()0%.Therefore

Therefore, 10 grams of 2.5% hydrocortisone cream should be mixed with the base to obtain the 1% cream.

98. Let x represent the balance on the card with the 1.75% interest rate. Then the balance on the other card is 600. x The total interest for the month will equal the sum of the interest for each card.

Group Activity Working with Real Data

5. Because the sheet of foil weighs 5.4 grams, from the previous problem the volume of the foil is 3 2 cm. Then, insert this information into the volume formula .Vlwh = Thus,

Copyright ©2013 Pearson Education, Inc