Addition with the Golden Beads & Stamp Game

Addition with the Golden Beads

and Stamp Game by ROBIN HOWE

ontessori children work with hands-on learning materials that make abstract concepts clear and concrete. e materials allow young students to develop a clear inner image of concepts in mathematics, such as value and the ability to conceptualize how big a thousand is, what we mean when we refer to the ‘hundreds’ column, and what is actually happening when we divide one number by another. is approach makes sense to children. Developed by Dr. Montessori over one hundred years ago, today’s research consistently shows that this 'innovative’ approach works exceptionally well with most children. As an example, let us consider the decimal system, which forms the basis of mathematics: units, tens, hundreds, and thousands. Normally, young children nd it di cult to understand or operate with quantities larger than twenty. ey cannot grasp the value of a hundred, thousand, or million ... much less the idea that one thousand is equal to ten hundreds or one hundred tens.

Montessori overcame this obstacle by developing concrete, tangible materials that not only represent values but are equal in scale, representing each value of the decimal system. Units are represented by single 1-centimeter beads; a unit of 10 is made up of a bar of 10 unit beads strung together; hundreds are squares made up of 10 tenbars; and thousands are cubes made up of 10 hundred-squares. Each one of these corresponding materials is presented to the child in a manner that shows how ten of each is equal to one of the values of the next place value: 10 units are equal to 1 ten; 10 tens are equal to 1 hundred; etc. Because the corresponding values are constructed from smaller values, they are to scale in weight and size.

Together, they form a visually and intellectually impressive tool for learning. Great numbers can be formed by very young children. Able to identify each value and its corresponding bead material, the preschool age child can actually build numbers by playing the Bring-Me Game. “Please bring me 3 thousands, 5 hundreds, 6 tens, and 1 unit.”

From this foundation, all of the operations in mathematics, such as the addi-

tion of quantities into the thousands, become clear and concrete, allowing the child to internalize a clear picture of how the process works.

Often, two children will work together to construct and solve a mathematical problem. Using sets of numeral cards, each will collect a quantity for addition by deciding how many units, tens, hundreds, and thou e corresponding cards themselves follow a color code in which the cards, showing the units 1 to 9 are printed in green; the cards showing the numbers from 10 to 90 are printed in blue; the hundreds from 100 to 900 are printed with red ink; and the cards showing the numbers 1,000 to 9,000 are printed in green again because they represent units of thousands. As the child progresses in arithmetic, this color scheme continues into the millions, each hierarchy (or family) is represented by the green of units, blue of tens, and red of hundreds.

As the children construct their number, they will decide how many units they want in their number, nd the corresponding cards that represent that quantity, placing the created number at the upper right-hand corner of their work space. Next, they go to the ‘bank,’ or central collection of Golden Bead material and gather that number of unit beads that corresponds with the numeral cards selected. is process is repeated with the tens, hundreds, and thousands. By doing this, they are not only becoming familiar with place value and developing the skills of naming numerals, but they are developing the deeper understanding of the value of thousand, hundreds, tens, and units.

Next, the two addends are combined, which we call the operation of addition. Beginning with the units, the children count the combined quantities to determine the result of adding the two together. If the result is nine or lower, they simply nd the large numeral card that represents the answer.

As the children count, if the quantity in the column is ten or greater, they stop at the count of ten and exchange them for the next highest quantity.

For example, in this case, when the units from both addends were combined, we had 13 units. e child gathers 10 units and carries them back to the bank to exchange them for

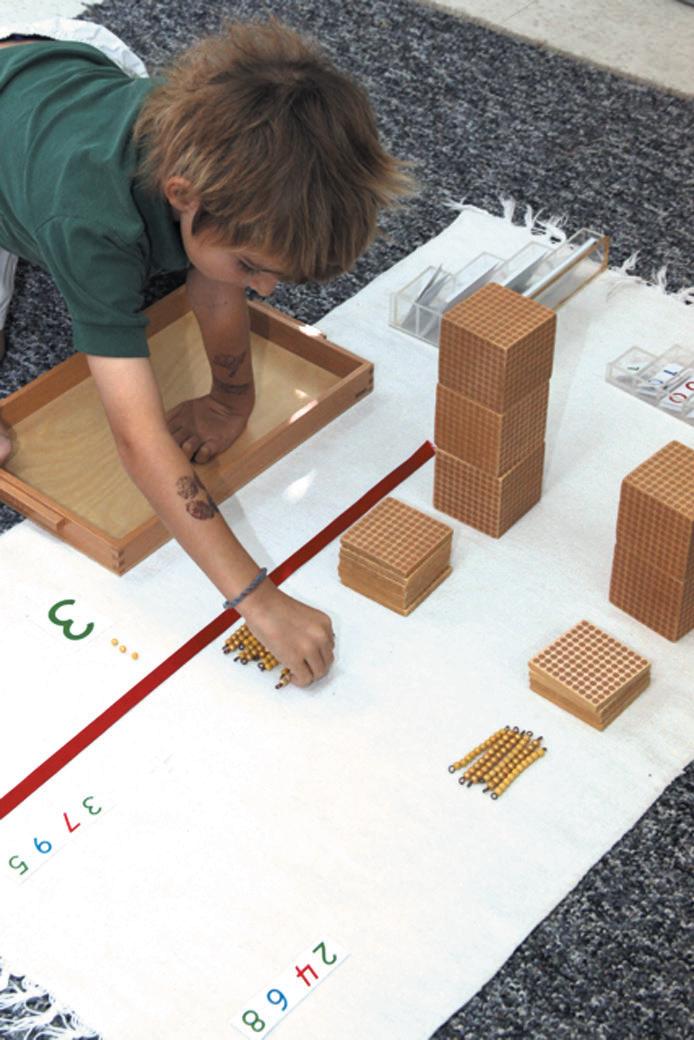
 above Working with his teacher, this young man has chosen to construct and add two quantities of Golden Beads. The fi rst is made up of 2 ‘thousand’ cubes, 4 ‘hundred’ squares, 6 ‘ten bars,’ and 8 ‘unit’ beads, equalling 2,468. The second is made up of 3 ‘thousand’ cubes, 7 ‘hundred’ squares, 9 ‘ten’ bars, and 5 ‘unit’ beads, totalling 3,795.

The two addends are placed on a rug with their corresponding numeral cards. Both number and material are named and created with the thousands (or highest place value) on the left. Right from the start of their education, Montessori children learn the concept of the units (ones), tens, hundreds, and thousands columns, and the way to read such large numbers.

The Stamp Game

a ten-bead bar: 10 unit beads is equal to 1 unit of ten. He has also used the large number cards to show the remaining number of units, which is 3.

is process is repeated with the ten’s column. Here, for example, we added 6 tens and 9 tens, plus the additional 10 that we carried forward. is gives us 16 tens. Our young friend gathers 10 ten-bead bars and takes them to the bank to exchange for another hundred square, which he places in the hundreds column. Finally, the child selects the correct numeral card to represent the total of the remaining ten bars, which is 6 tens.

e process is continued with the hundreds and thousands. Helping the children to understand that 10 tens is the equivalent of a hundred leads Montessori students to more easily grasp complex concepts in the addition of large quantities, subtraction, multiplication, and division. When everything is done, the children have their answer: 2,468 + 3,795 = 6,263.

Notice how the cards increase in length as we grow from units to tens, then hundreds, and thousands. When the cards are laid down with the thousands on the bottom, each of the columns is shown in the answer: 6 thousands, 2 hundreds, 6 tens, and 3 units.)

Once the children have mastered the Golden Beads, they progress into more abstract materials that represent value.

A favorite of Montessori students and parents alike is the Stamp Game that has small 2cm wooden stamps that represent each value. Like the cards used with the Golden Beads, the stamps follow the same color scheme: green for units; blue for tens; red for hundreds; and again green for thousands. is same color scheme is used with Montessori materials that the children will use later to nd square roots and in the study of algebraic concepts.

e students will perform the same operation as they did with the Golden Beads; however, because the stamps are the same size, this requires more abstract comprehension of the material. ey can use the Stamp Game on a smaller work space, creating both addends and then adding them, exchanging when the number of each place value is greater than nine. Like the Golden Beads, the children create the rst addend with the stamps, starting with the units and proceeding with the tens, hundreds, and thousands. After they have created their rst addend, they build a second addend, leaving a space to clearly separate the two addends. Once the two numbers are created in two columns for each place value of each addend, they are pushed together to make one value, representing the sum of the two addends.

Starting with the units, the student counts the stamps, recording the number. In the example shown, there were 10 units of tens, which need to be exchanged for 1 unit of a hundred. is yields a total of 9 hundred tiles in the hundreds column.

By this stage, in addition to constructing the answer with the number cards, most children will also record their work on large square graph paper. e nal sum of 2,468 + 3,795 = 6,263. Many parents and teachers think that, while the Montessori math materials are appropriate for children during the preschool and kindergarten years, when they go on to the elementary grades, children shouldn’t need them. In fact, as the children progress into ‘more di cult or advanced math,’ continuing to provide concrete opportunities for learning becomes even more important.

The Short Bead Stair

The Teens & Tens Board

 right Using the Golden Bead material, the child sees the numbers one through nine represented as individual units. But, as we mentioned earlier, although the child can count the beads one at a time, it takes many years before most children can recognize and really understand the idea of number except by one-to-one correspondence.

To help the child truly begin to grasp the idea of quantities from one through nine, Dr. Montessori prepared a set of colored glass beads, in which each quantity is represented by the appropriate number of individual beads wired together as a bar with a specifi c, easily recognizable color. In this material, a “1” is represented by a single red bead; a “2” by two green beads strung together; the “3” by three pink beads, and so on up through the ten Golden Beads that represent a unit of ten.

The children work with the Short Bead Stair for many years, using the material to add and subtract, carry, borrow, explore multiples, and for many other arithmetic processes. For example, to multiply 9 x 8, the children would lay out eight “9” bars or nine “8” bars. By counting the result, they can check their work.

 left This material is made up of two different sets of boards that children use to explore the nature of quantities and numbers greater than nine. Each set consists of two boards, which are laid in a vertical row. The two boards are divided into nine sections, each of which is fi tted with a thin frame into which the children can slide wooden cards on which the numbers 1 through 9 have been printed. Numbers have also been printed on the surface of the board, spaced so that when the cards are slid into the frame they will cover up one of the two digits. On the Teens Boards, the number 10 is printed in the nine spaces created by the frames. The children arrange the number cards from 1 to 9 in order, and slide them into the frames, creating the numbers 11, 12, 13... and so on through 19.

Using the “Ten Bead Bars” and the Short Bead Stair material described earlier, the children lay out the numbers 11 through 19 concretely. For example, the number eighteen would

Elementary students rarely have the ability to think abstractly. ings that are not connected to their own concrete experience tend to be confusing. Mathematics is inherently abstract. Montessori’s objective is to use these concrete mathematical materials to help children develop an inner picture of mathematics that will last a lifetime.

Montessori often used the analogy of the purpose of preparing a smooth runway for an airplane. Once the plane has gained enough speed to y, it can travel in any direction at great speed. However, the problem is how to provide a safe, smooth environment in which the airplane can begin the transition from a stationary object on the ground to a soaring thing of ight.

e runway is the prepared environment essential for the aircraft. e Montessori math materials re ect an attempt to prepare an environment in which children can come to understand mathematics and begin to think and operate mathematically. ¢

be formed by placing one ten bar in the tens column and one brown eight bar in the unit column. This gives them a very clear picture of how the teens are formed and written: ten and one is eleven, ten and two is twelve, etc. On the Tens Boards, the numbers 10, 20, 30, 40 through 90 are printed in the nine spaces created by the frames. They use the individual number cards to form numbers in the tens, such as 53, 24, 79, etc. and use the Golden Bead tens and unit beads to build their concrete representations along side.

The Hundred Board

 above The Hundred Board challenges the young child who can count aloud from one to one hundred to lay out the numerals in the same sequence. The Board is a square divided into ten rows with ten small squares along each row. The children work with a set of one hundred wooden tiles that are labeled from one through one hundred.

Students spread the tiles out on the rug, arrange them in numerical order,and place them, one tile at a time, on the Hundred Board, working from the upper left-hand corner along each row to the right, down to the next row, and so on until complete.

When they are comfortable with this, they attempt the same exercise by fi lling in the squares on a blank chart drawn to duplicate the surface of the Hundred Board.

The Square & Cube Chains

  above & right Following the same concept, the Square and Cube Chains introduce the child to the concept of skip counting by ones, twos, threes, fours, etc. through tens. Each chain is constructed by connecting multiples of the Short Bead Stair, using the same color scheme that the children learned before: red “units,” green “2” bead bars, pink “3” bead bars, etc. The material also introduces the children to the concept of the squares and cubes of the numbers one through ten.

There are two chains for each number: one set representing the squares of the numbers one through ten, and the other representing the cubes. Thus, the square of fi ve is shown as a chain of fi ve “5” bead bars (5 squared = 25) and the cube as a chain of twenty-fi ve “5” bead bars (5 cubed=125).

The material also includes a set of bead bars connected to show the squares and cubes of the numbers as actual squares and cubes. The children use the bead chains to skip count, working with number arrows similar to those used with the Hundreds and Thousand Chains.

 above The “bank” is a name given to a collection of Golden Bead materials, which includes enough “units,” “10” bars, “100” squares, and “1,000” cubes to allow several children to create large numbers. In one of the fi rst exercises, the children explore the equivalencies of the decimal system. They learn that ten “units” can be exchanged at the bank for a “10” bar, and that a “10” bar can be exchanged for ten “units.” They also fi nd that ten “10s” can be exchanged for a “100” square, ten “100s” for one “1,000,” and that each can, in turn, be broken down into its equivalent in the smaller quantity.

Using the Golden Bead material, the children can build two or more large numbers and add them together. By going through the steps of addition in this very concrete manner, the children have a clear impression about what addition means. They also come to understand the process of exchanging, as they count the new quantities in each of the columns and trade in groups of ten “units” for one “10” bar, which they place in the “10s” columns; ten “10” bars for one “100”; and ten “100s” for one “1,000.”

Once they understand how to add with the Golden Beads, Montessori children begin to use them to multiply, subtract, and divide. For example, to divide the quantity 3,333 by three, a child would set out three wooden skittles, and, beginning with the largest quantity, in this case the “1,000” cubes, he gives one “1,000” to each skittle. He continues on with the “100s,” “10s,” and “units.”

If the child were challenged to divide this same quantity by four, he would begin by exchanging the three “1,000s” for thirty “100s” squares, add them to the stack of three “100s,” and then he would distribute them equally. After placing eight “100s” beneath each of the four skittles, he exchanges the remaining one “100” for ten “10” bars. This process of exchanging continues until the fi nal answer is derived: eight “100s,” three “10s,” three “units,” with a remainder of one “unit.”

The Bank Game

Multiplication & Division

The Short Multiplication and Division Boards o er other pathways to abstraction with these two operations.

 left Using the Multiplication Board children lay out individual unit beads on a board organized into nine rows of nine shallow holes. They lay out the beads in rows. For example, 4 x 8 would be eight columns of four beads per column.

 right The Division Board is similar, except that here each vertical column of shallow holes represents one equal share, where a quantity is divided into two or more groups. Children place one small skittle at the top of each column to mark the number of shares that will be in their divisor; to divide 24 by 6, they begins by placing 6 skittles along the top. Then they count out the number of beads that they want to divide and begin to distribute them, placing one bead in each hole from the top left to right and then down a row, until they fi nally have shared their quantity equally among the vertical columns. Any beads left over are their “remainder.”

MONTESSORI MATH

The Fraction Circles & The Fraction Skittles

The Snake Game

As the children become more and more comfortable with the Golden Beads, they eventually begin to ask whether there is anything smaller than the unit. The Fraction Skittles, and many experiences in the classroom, gradually introduce them to the concept of a quarter, half, and whole.

The Fraction Circles take this concept much further. It is a set of ten metal frames into which are set ten circles: one left intact; one divided into two parts; another into thirds, fourths, fi fths through tenths.

The children learn the terminology, how to write fractions out as fi gures, and begin to explore the concepts of equivalence (2/4 = 1/2) and basic operations with fractions (1/2 + 2/4=1).

The Snake Game helps students to learn how to quickly make exchanges among different quantities that make up the number ten and higher. The game is played by laying out a snake using the colored bead bars (which represent the numbers 1 to 9 as rods of colored bead bars, with each quantity represented by beads of a different color). The children begin counting from the start of the chain, noting when they reach the number 10, and removing the beads that have been counted.

At this point, they need to replace the counted beads with a Golden Ten Bead Bar and add in a new bead bar that represents the quantity left over. Let’s imagine that the fi rst 3 beads in the “Snake” were a 5 bead bar, a 3 bead bar, followed by a 6 bead bar. When the child has replaced these 3 bead bars with a 10 bar, the original beads added up to 14. To leave the same beads in the Snake as where they were at the start, they add a 4 bead bar after the golden 10 bead bar that they just laid down. They continue replacing all the beads in the Snake with Golden Bead Bars, until the Snake has been turned from many colors to one that is completely golden, except for any quantity that remained.