Fairness of Dice

Text: Emile van der Veer

Introduction

For a really long time now, I have been interested in shapes and dice. These two subjects fit really well together. For example, one can ask themselves which shapes can make ‘fair’ dice. Where we call a die fair if there is equal probability for each number to be the result of a roll of a die. There are a whole bunch of trivial options to obtain such a result, like putting the same number on every face, or making a die with only face. However, this is not really useful if you want an actual physical die.

Symmetries

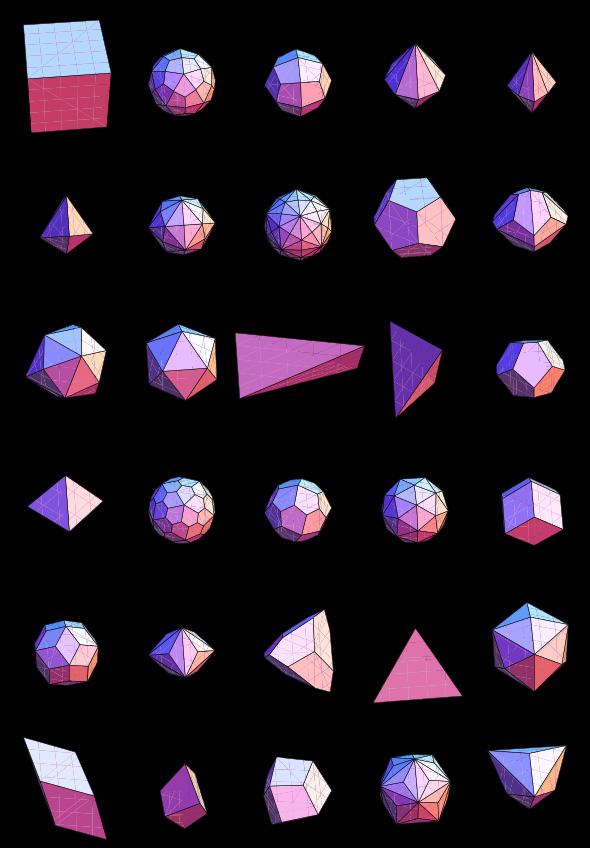
As it turns out, for a die to be fair, we need certain amounts of symmetry. The easiest option for 3-dimensional shapes with maximal symmetry is to take the five Platonic solids: the tetrahedron, the cube, the octahedron, the dodecahedron and the icosahedron. These five shapes are indeed the most used shapes for dice, with the regular six sided die of course being the cube. However, these five shapes are not the only shapes that could make for fair dice. For example, a coin is a really good example for a two sided die. Although, there is an all so small possibility that the coin will land on its side, making it not completely ‘fair’. In theory we can fix this by making the coin infinitely thin, but this does not really hold up in practice. In practice, it is much easier to define a coin to be a fair two sided die if we simply re-toss the coin if it lands on the side, until it lands on an actual ‘valid’ face. All these shapes could work as fair dice, but these are not the only shapes that could work as fair dice. Actually, all the shapes that fall under the family of the Isohedra can be used to construct fair dice. They do not have maximal symmetry, but they all have enough symmetry to produce fair dice.

Regular Polyhedra

The platonic solids work as fair dice because they are really symmetrical, they have symmetry over all axes orthogonal to their faces, around all vertices and edges. This makes them regular polyhedra. If we go to 2D, we can very easily find an infinite list of regular polygons (the 2D equivalent of a regular polyhedron), a small example to illustrate this can be found on https://www. geogebra.org/m/jjmxy9xz. Using this we can easily construct the five Platonic solids. However, these five are not the only regular polyhedra, they are merely the only five regular convex solid polyhedra. If we allow polyhedra that are not solid, we cannot make dice out of them, but theoretically we should be able to make dice out of shapes that are not convex. Deciding which number actually comes up might be a bit tricky, but it should be possible. If we allow this, we are able to add four more regular polyhedra to our list, namely the Kepler-Poinsot polyhedra. A nice video about this can be found on the YouTube channel jan Misali, and is titled “there are 48 regular polyhedra”.

Conclusion

Most of the time, the dice that are made are cubic dice, but quite some games need a four-sided, eight-sided, ten-sided, twelve-sided, twenty-sided and onehundredsided dice as well. These are often made using the Platonic solids and two pentagonal trapezohedra. But we could also construct fair dice out of a whole lot of other shapes in the Isohedral family. And there actually are dice made out of these shapes, this has allowed people to make dice with ‘weird’ amount of faces, like a 5 sided die, or a 30 sided die. But other examples of dice have also been made that aren’t even in the family of Isohedral figures that rely on different kinds of rules on what counts as a die. You could for example make a prism out of a regular polygon and only roll it over the axis connecting the middlepoints of the regular polygons. This is not a shape that is part of the Isohedral family, but it would still make for a perfectly fine die. And then you can also look at a whole bunch of other factors that determine how fair a die is, like the weight distribution and the number configuration on the dice, but most of these can be considered to not produce significantly different re- sults unless one makes the die unfair on purpose.